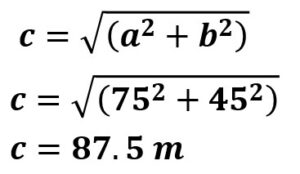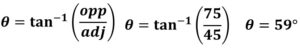Learning Targets
- I can to combine 2D vectors that lay on an X or Y axis to determine a resultant
- I can to combine 2D vectors that don’t lay on an X or Y axis to determine a resultant
- I can describe the resultant including a magnitude, angle, and description
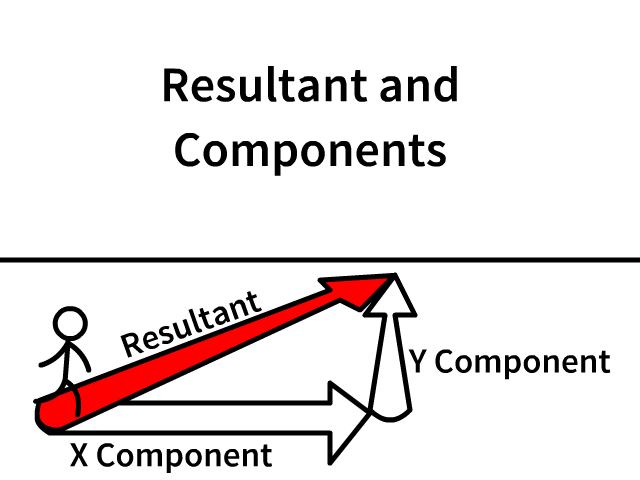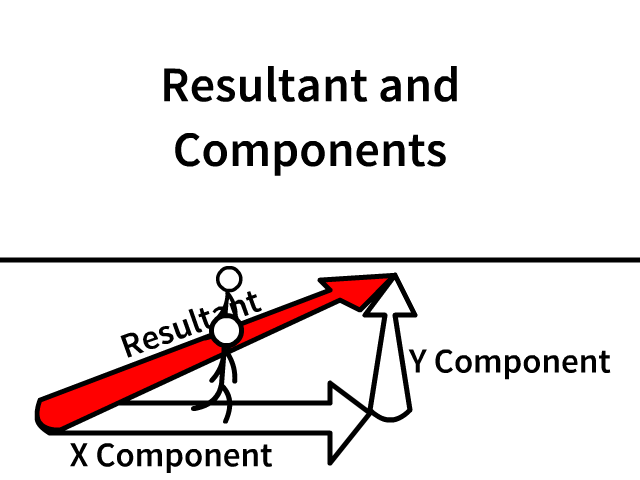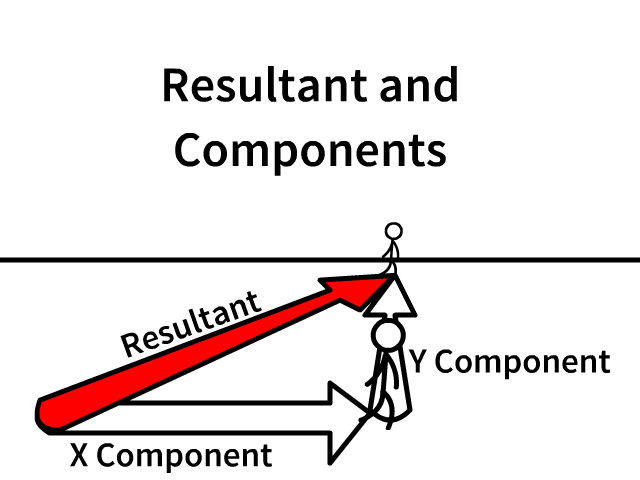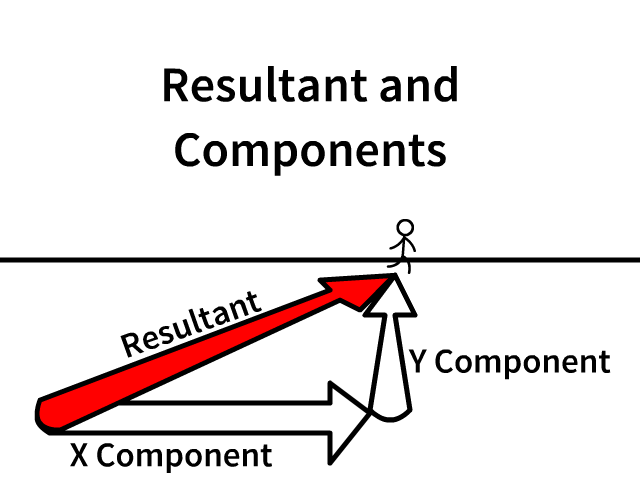
Resultant and Components
The resultant is the overall vector
Components are individual parts which create the resultant
- Following the individual components lead you from the origin (staring point) to the same end
- Component arrows are always draw in the direction that would lead you from the same start to the same end
In easier problems the components lay along the X and Y axis. In longer problems you start by breaking down vectors at angles to their X and Y components.
Observe the animation how the stickman is in the same position along the X or Y axis the whole time. The animation is following the X axis and then the Y axis. In reality the X and Y components are happening at the same time as the overall resultant.
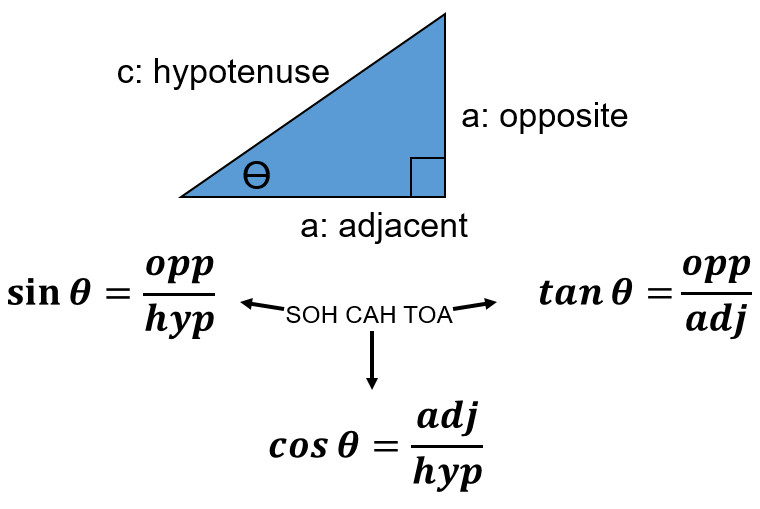
Trigonometry Reminders
Before we go any further be sure to remind yourself about how to solve for sides or angles of a right triangle. Also double check that your calculator is in degrees and not radians mode.
- The opposite of a triangle (a) and adjacent (b) change based on the location of the angle in question
- The hypotenuse is always the longest side
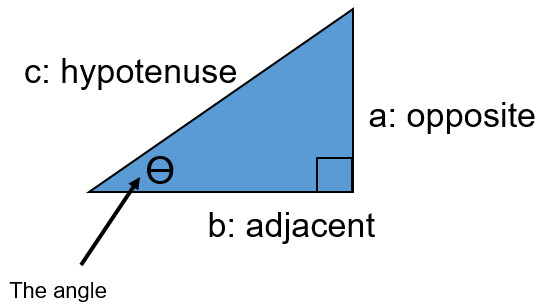
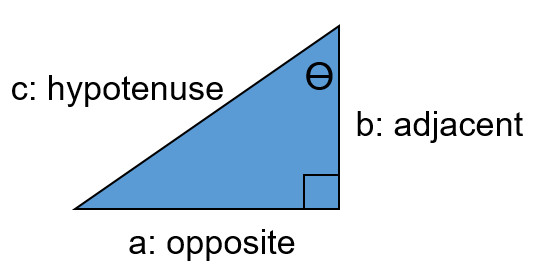
To determine a side with a right triangle when you know two other sides
- Use Pythagorean Theorem
a² + b² = c²
To determine an angle with a right triangle when you know two sides
Use what commonly is referred to a SOH CAH TOA
Calculator Check Time
Anytime you are going to be typing in Sin, Cos, Tan, etc. into a calculator and using degrees, be sure your calculator is on the degrees mode

Combining One X-Axis and One Y-Axis Vector to get a Resultant
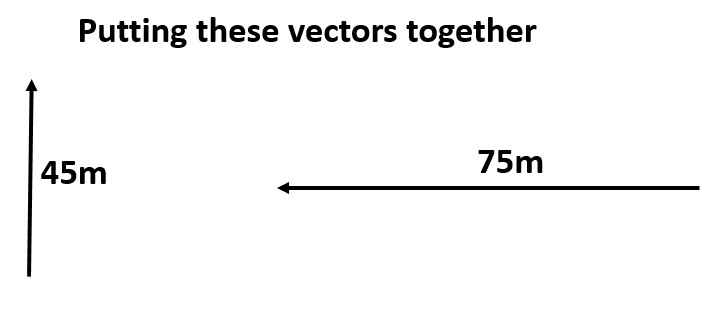
When adding two vectors on different axis, you can't simply add or subtract.
Follow the following steps to add vectors and get a three part magnitude, angle, and description of angle resultant answer.
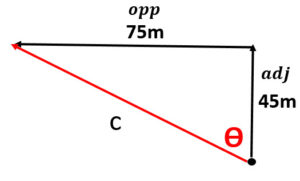
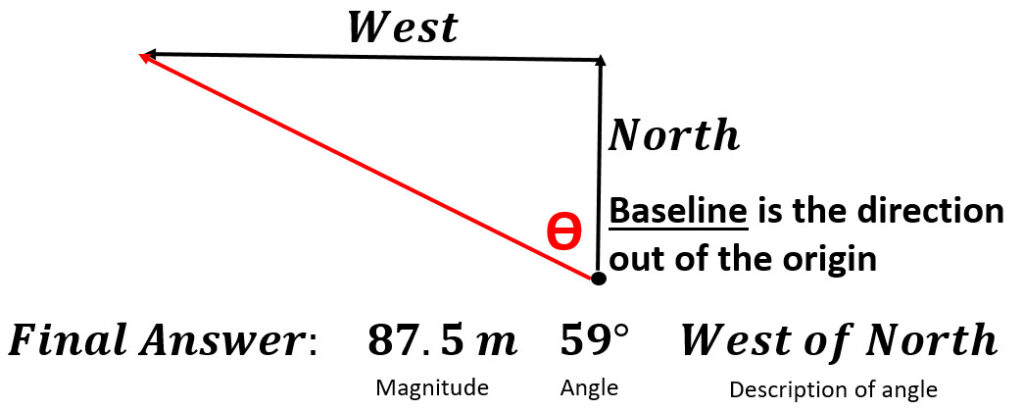
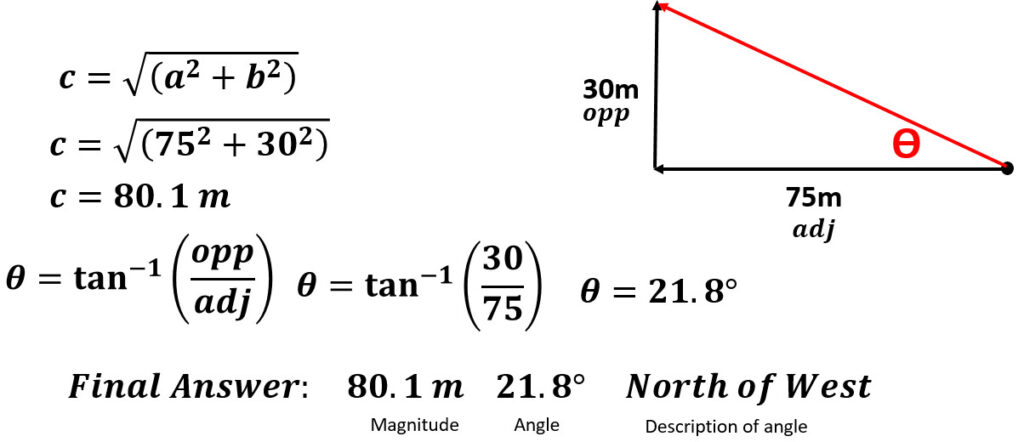
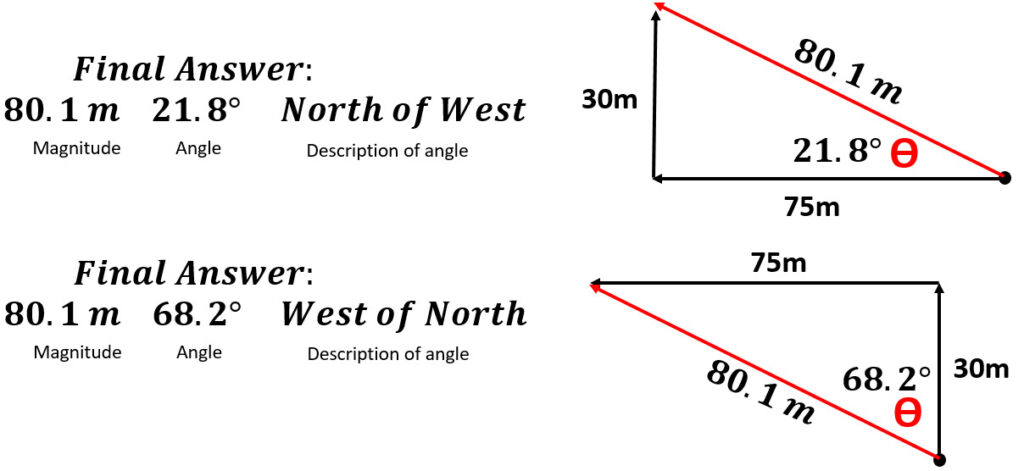
Example: What is the resultant of a horse that travels 45 meters north followed by 75 meters west?
1. Draw an origin dot
2. Draw the first component (45 meters north) and label it
3. Draw the next component (75 meters west) and label it starting at the previous arrow tip
4. Go back to the origin and draw the resultant from beginning to where the components ended.
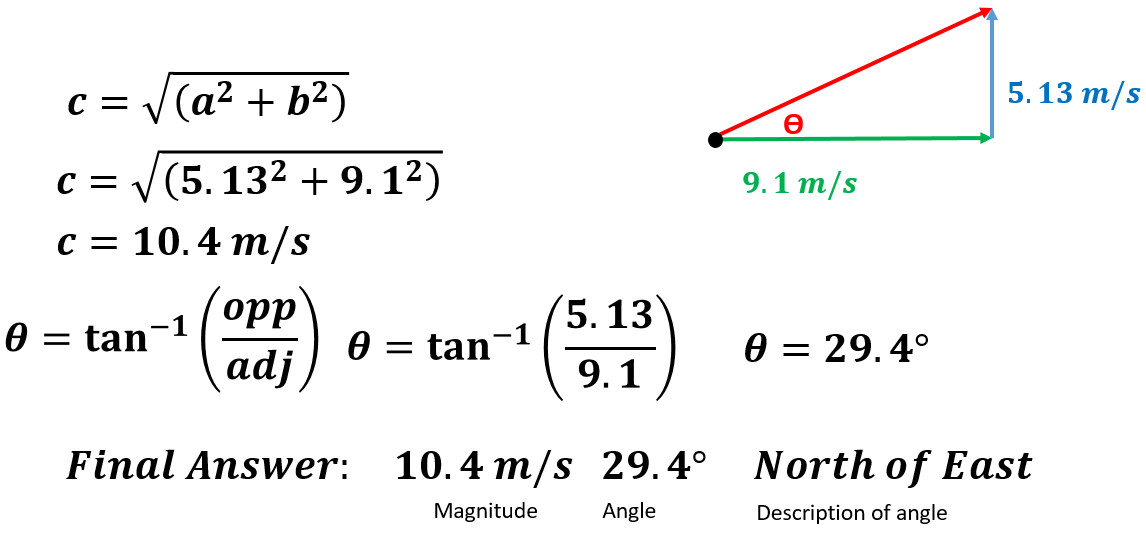
5. Use the Pythagorean Theorem to determine the hypotenuse.
6. Use inverse tangent to figure out the angle.
7. Describe the angle ____ of ____. The last direction is the baseline (line coming out of the origin dot) so W of N.
Practice:
Q1: What is the result of flying 95 m/s north with a 10 m/s breeze blowing east?
Combining More than two X-Axis and Y-Axis Vectors to get a Resultant
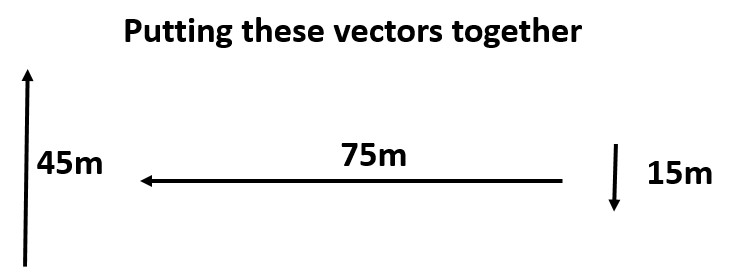
Example: What is the resultant of a person that travels 45 meters north, 75 meters west, and 15 meters south?
- Start by putting any X components together as we did in the 1D motion section
- Here you only have a 75 m west component
- Then put the Y components together as we did in the 1D motion section
- Here you have a 45 m north and 15 m south you will have to put together
- Now draw an origin dot and use the resulting X then Y components
- Then draw your resultant from origin to finish
- Solve for the resultant magnitude, angle, and description of angle as we did in the prior problems
Combining an off Axis Vector and One on Axis to get a Resultant
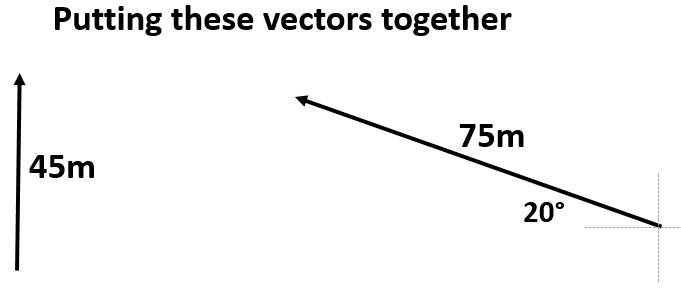
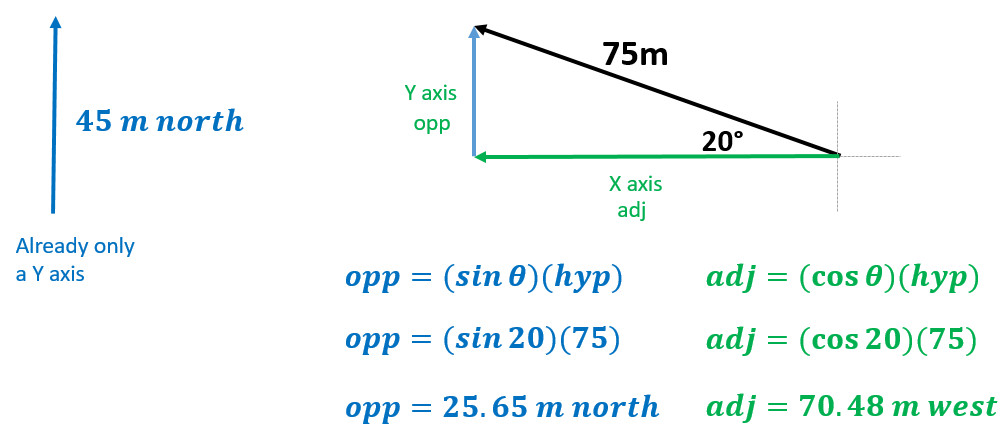
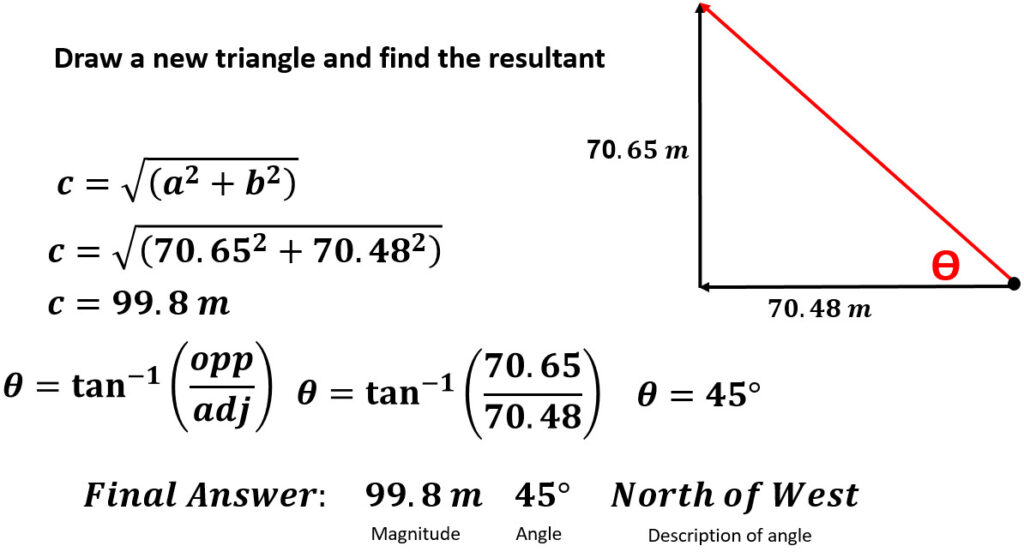
Example: Where is Joe located after traveling 45 meters North followed by 75 meters 20° North of West?
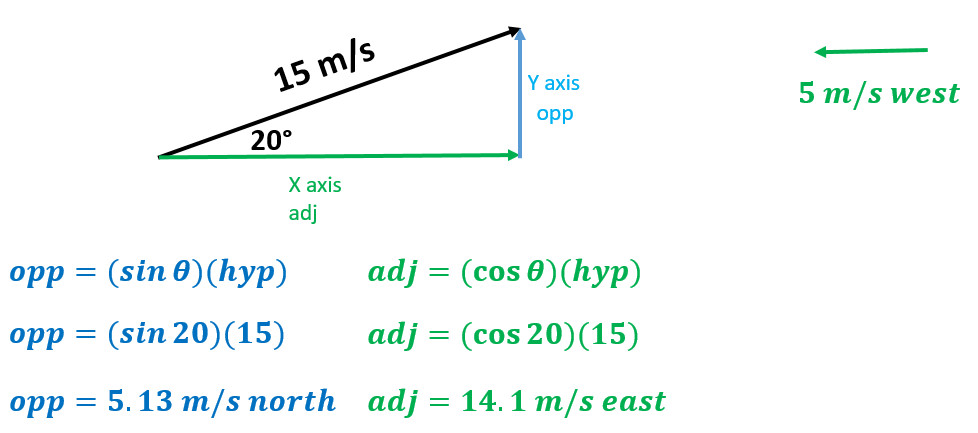
- Start by finding the X and Y components of any off axis vector
- Here find the X and Y components of 75 meters 20° North of West
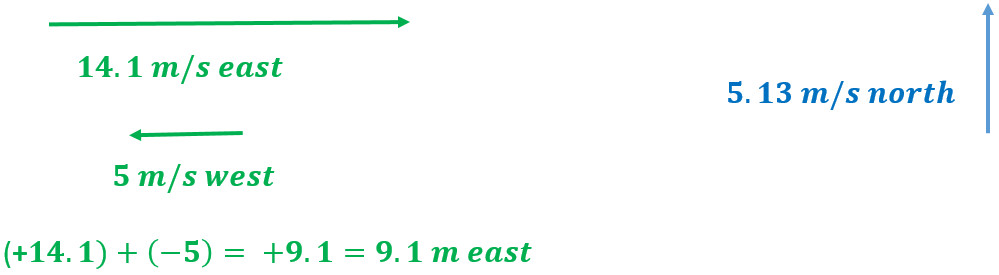
- Put any X components together as we did in the 1D motion section
- Here you only will have one X component
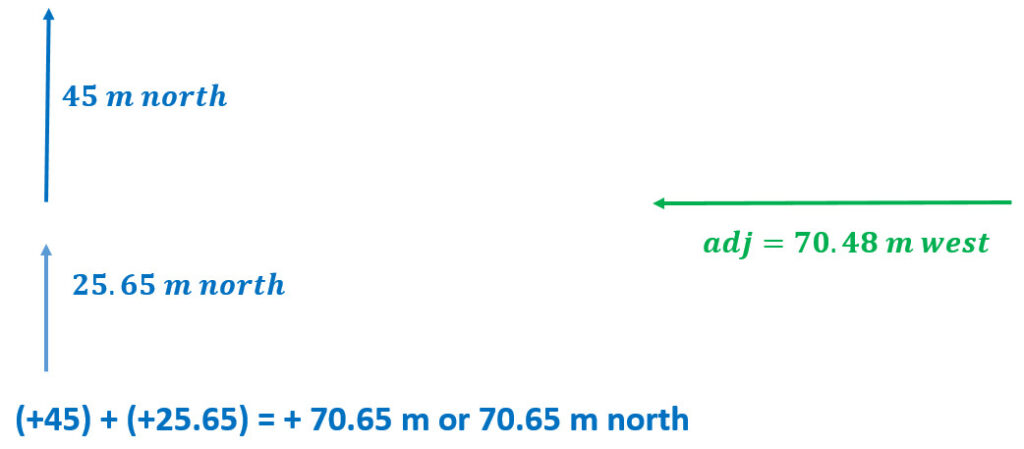
- Put any Y components together as we did in the 1D motion section
- Here you have two Y components to put together
- Now draw an origin dot and the resulting X then Y component
- Then draw your resultant from origin to finish
- Solve for the resultant magnitude, angle, and description of angle
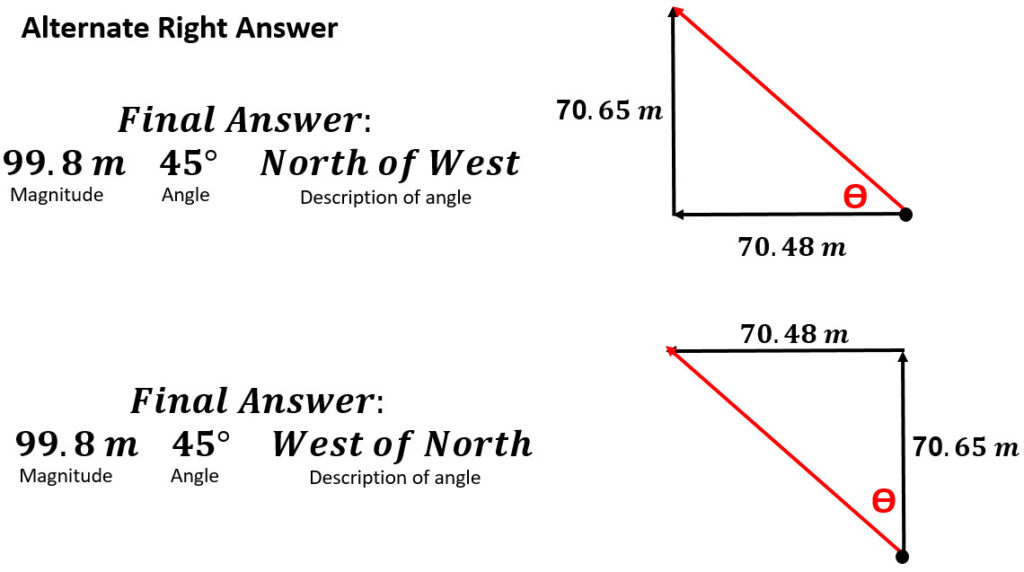
- Alternative right answer if you drew the north component before the west
Practice:
Q2: What is the result of boating 15 m/s 20° North of East with a 5 m/s current to the west?
Combining Multiple off Axis Vectors to get a Resultant
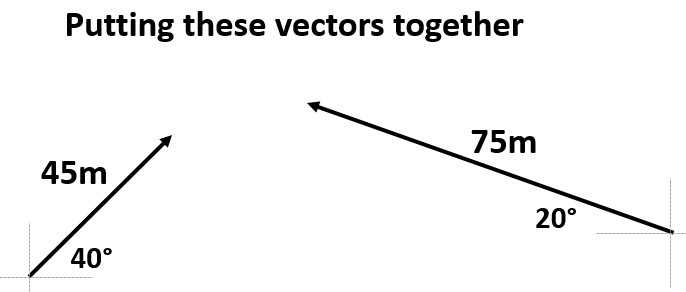
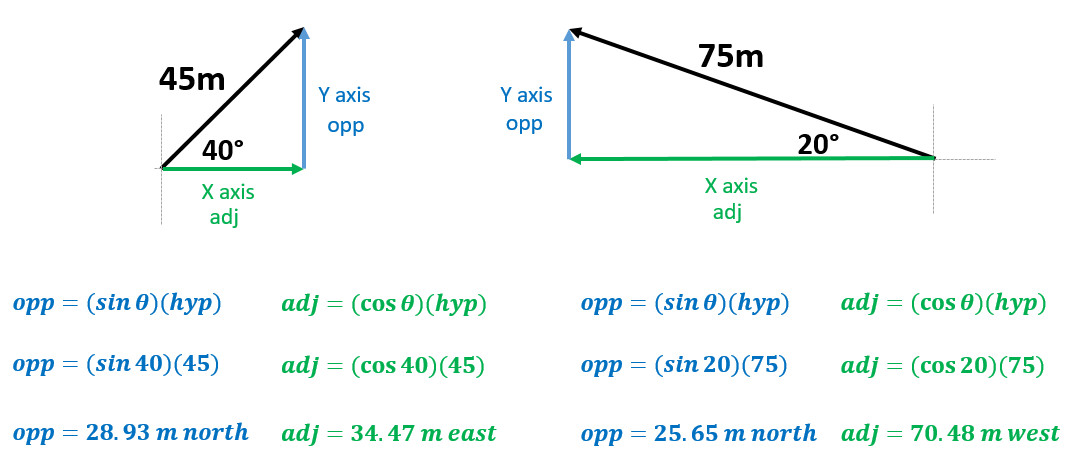
Example: Where is Joe located after traveling 45 meters 40° North of East followed by 75 meters 20° North of West?
- Start by finding the X and Y components of any off axis vector
- Here find the X and Y components of 45 meters 40° North of East
- Here find the X and Y components of 75 meters 20° North of West
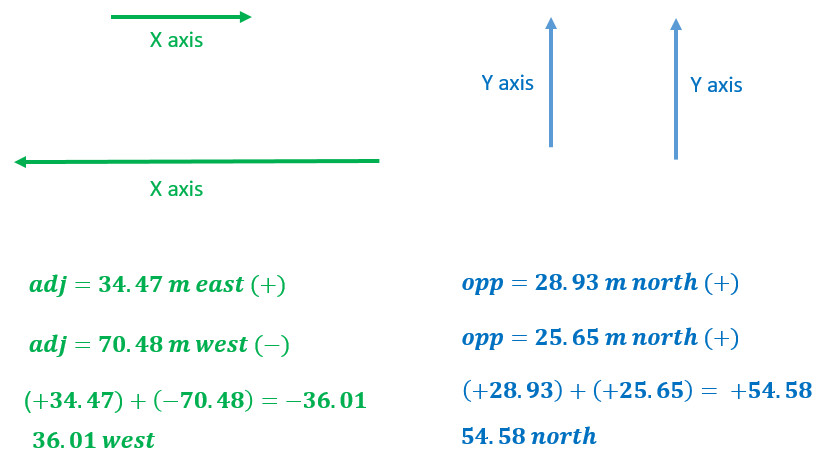
- Put any X components together as we did in the 1D motion section
- Put any Y components together as we did in the 1D motion section
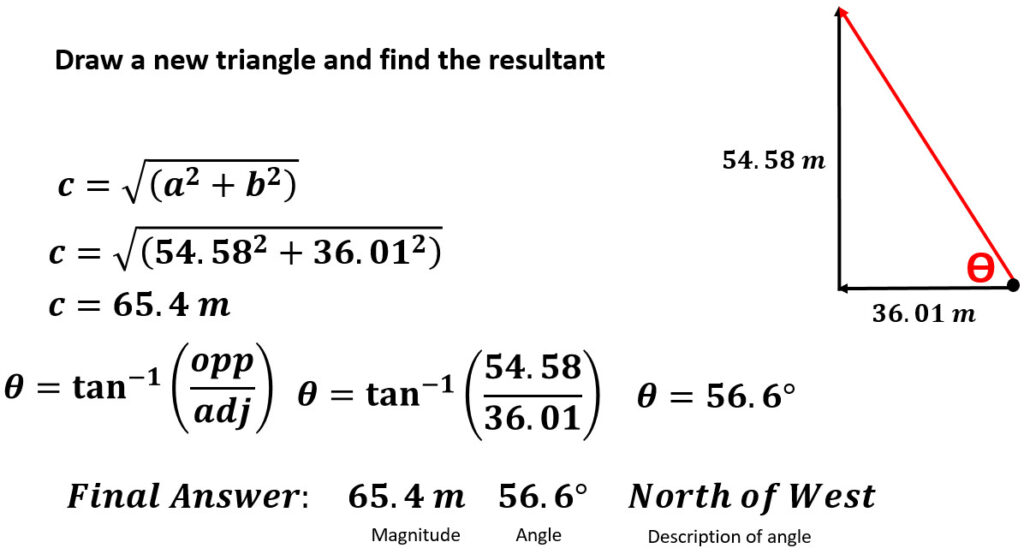
- Now draw an origin dot and the resulting X then Y component
- Then draw your resultant from origin to finish
- Solve for the resultant magnitude, angle, and description of angle
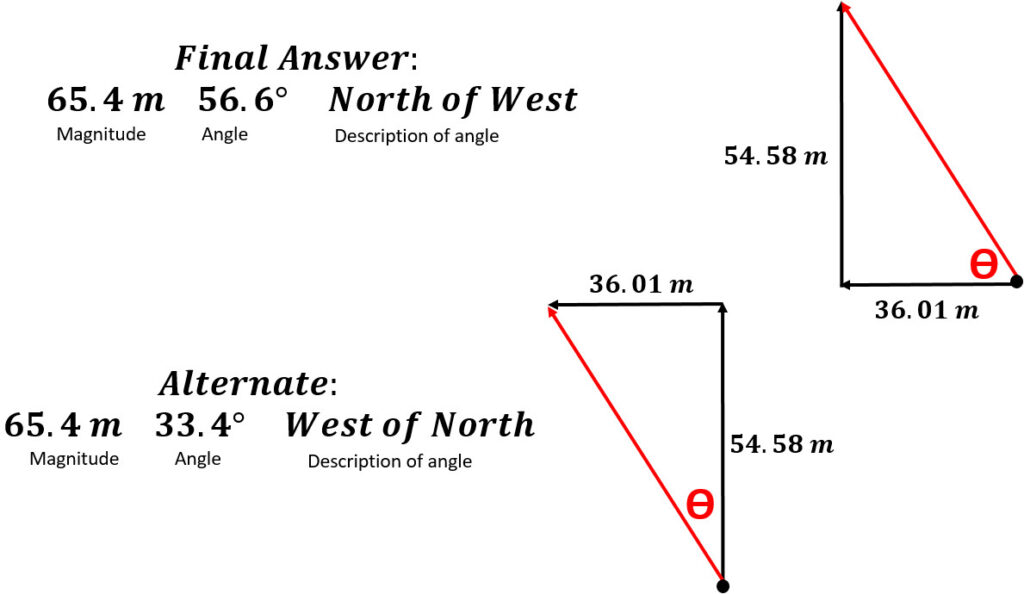
- Alternative right answer if you drew the north component before the west
Problem Set:
Finish the practice problems from this unit found below. You can use the video here to see solutions and check the answers.
(Q1 and Q2 were done in the lesson earlier)
Watch the video here for help on solving these problems
Find the resultant of the following vectors
Q3: 25 m north and 68 m north
Q4: 43 m east and 70 m north
Q5: 158 m west and 40 m south
Q6: 60 m/s south and 85 m/s east
Q7: 92 m/s north and 18 m/s west
Q8: 60 m/s north and 40 m/s 20° S of E
Q9: 92 m/s north and 25 m/s 40° N of E
Q10: 88m directed 22° N of E and 41 m directed 52° S of E
Q11: 35m directed 52° N of E and 67m directed 47° W of N
2D Non Projectile Motion Quiz
Links
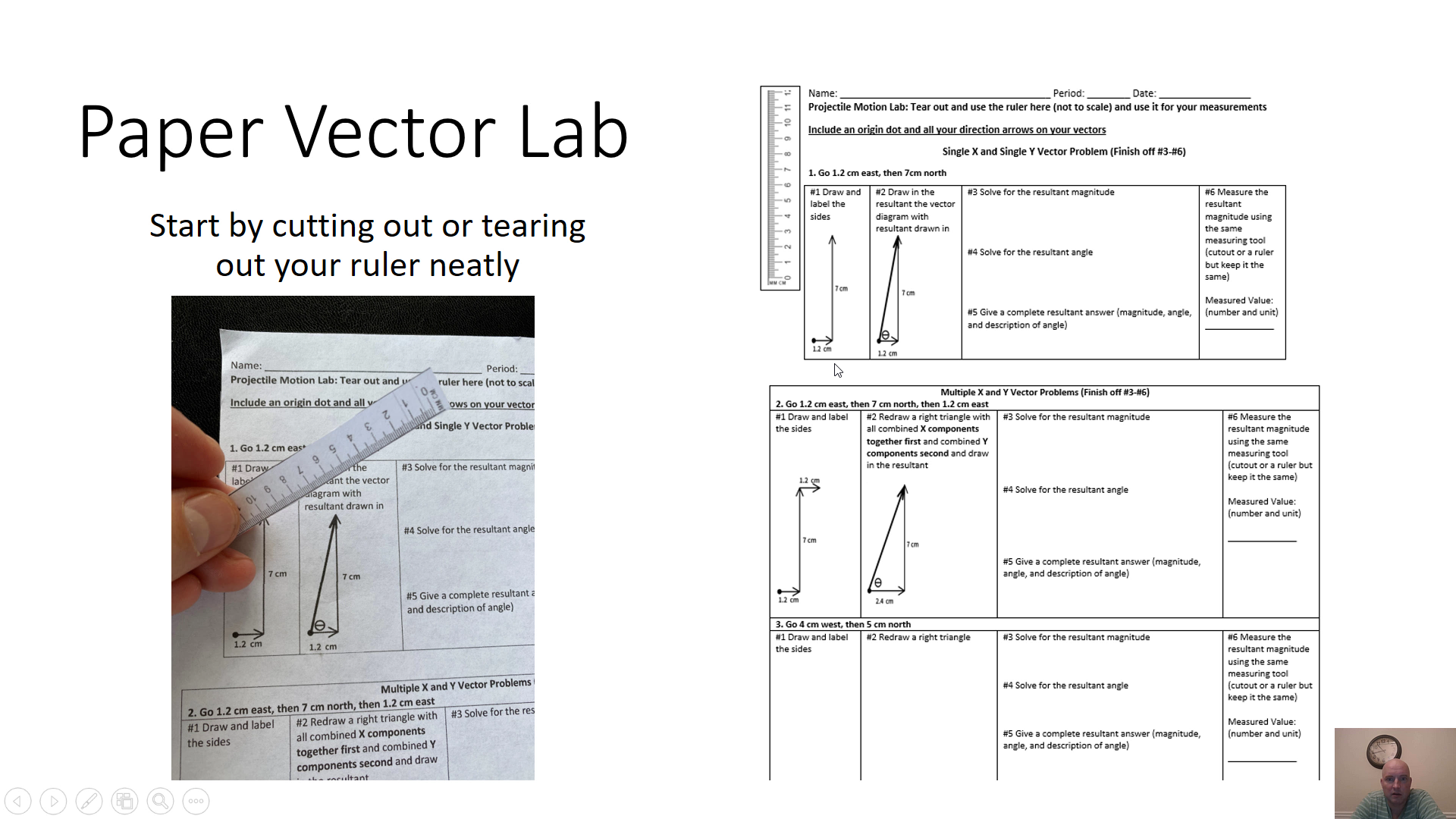
- Paper Vector Lab
- Continue to part 3: Projectile Motion
- Back to the Main 2D Motion Page
- Back to the Stickman Physics Home Page
- Equation Sheet