Introduction and Unit Analysis
Unit Analysis Learning Targets
- I understand the difference between a variable, unit, and equation
- I can do a basic metric-nonmetric and metric-metric conversion
- I can write into and out of scientific notation
What is Physics?
Physics is the study of the physical world and natural phenomenon.
Kinematics - A branch of physics that studies of motion.
Many problems require you to seek out information so you can answer a question.
- How far?
- How long?
- How fast?
- How much faster?
For example this question:
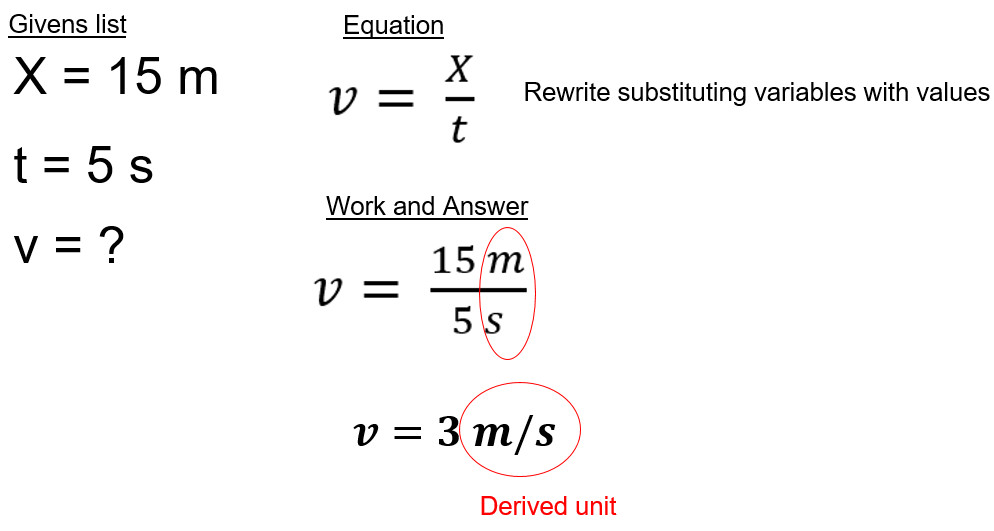
- How fast are you traveling if you travel fifteen meters in five seconds?
Here we are given two knows and one unknown and can begin to create a solvable problem.
| Question | Variable | Number and Unit Abbreviation |
| How far? | x | 15m |
| How long did it take? | t | 5s |
| How fast? | v | ? |
Next we would find an equation that has the knowns and unknowns in it, which leads you to the equation v=x/t
After we plug in the values and solve we find the answer to be 3 meters per second or 3 m/s with an abbreviated unit.
The unit m/s was derived by the units for the variables x and t as seen in the picture.
MKS System
In physics we use a system of units called MKS derived from the common metric SI units which stands for system international.
What MKS Stands For:
- M: Meters
- K: Kilograms
- S: Seconds
Since physics deals with large objects like planets, instead of grams, like in chemistry, we use kilograms as a standard unit of mass.
Converting
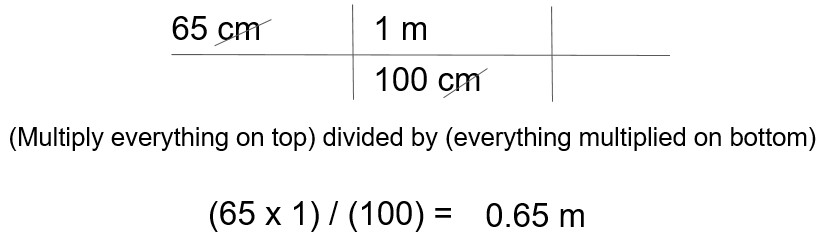
If the units given are not in the MKS units we will often have to convert.
Non-Metric to Metric Conversion
Lets say you were 652 miles away from Atlanta and you were asked to convert this to meters, you would need a conversion ratio.
- 1 mile = 1609.34 m
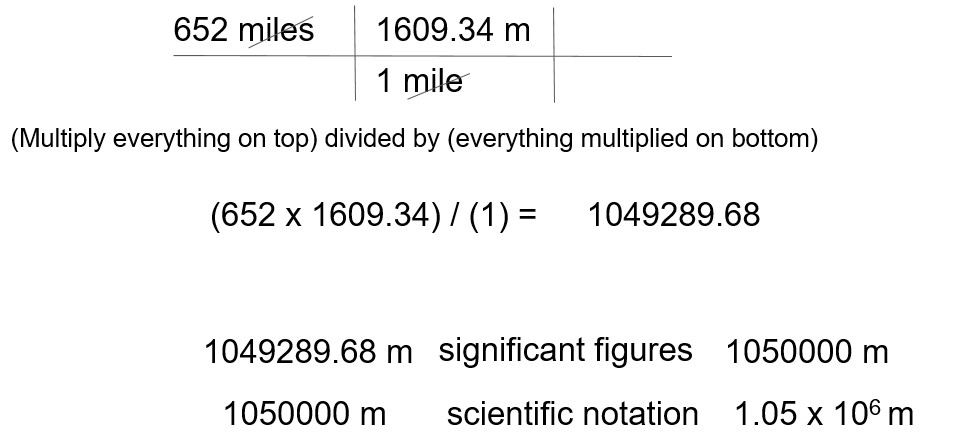
After converting, we would have to think about significant figures and putting the number into scientific notation as you see in the picture.
Metric to Metric Conversions
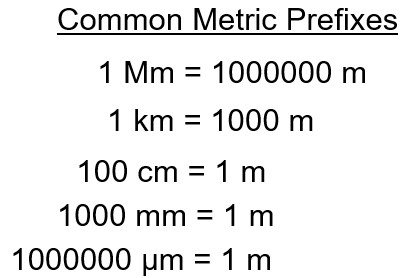
In science we are measuring in meters and often have a prefix like centi- in front of the unit meter which represents a factor of ten.
Look at a few common prefixes we use in physics which will help you convert.
Let assume you measured your desk and found it to be 65 centimeters and you needed that measurement in meters for your lab.
You would have to use the conversion 100 cm = 1 m
The conversion would then look as follows:
Here is another example:
Deimos, a small moon of Mars, has a radius of 11.265 kilometers. How many meters is this?
After converting you would find that 11.265 km equals 11265 m.
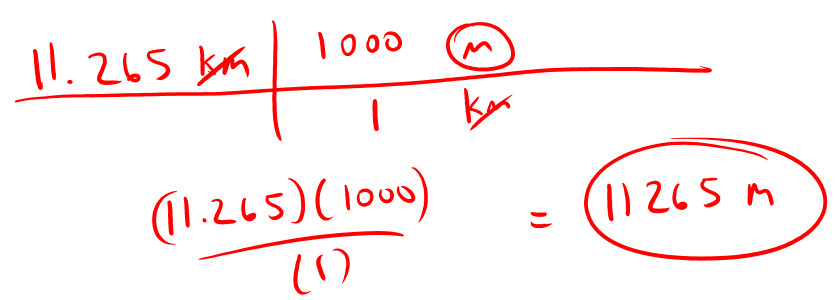
How to Write Into Scientific Notation
When Starting With a Big Number
- Rewrite the number with only one digit to the left of the decimal point and dropping off the zeros after the last number and add a (x 10)
- If the new number is smaller than the original, count how many decimal places and add that as a positive exponent
654000000 becomes 6.54 x 108
When Starting With a Small Number
- Rewrite the number with only one digit to the left of the decimal point and dropping off the zeros after the last number and add a (x 10)
- If the new number is bigger than the original, count how many decimal places and add that as a negative exponent
0.000341 becomes 3.41 x 10-4
Question: How do you write 0.0051 in scientific notation?
How to write out of scientific notation
Negative exponent example: 3.41 x 10-4
If you have a negative exponent, drop the (x 10) and move the decimal that many spaces to the left
0.000341
Positive exponent example: 4.5 x 105
If you have a positive exponent, drop the (x 10) and move the decimal that many spaces to the right
450000
Question: What non-scientific notation number does 4.55 x 102 represent?
More Practice
Practice Problems
Convert into scientific notation
1) 4100
2) 0.00235
3) 3230000
Express the following numbers in scientific notation
1) 8.32 x 10-2
2) 5.4 x 104
3) 9.67 x 103
Convert the following numbers to meters with the correct scientific notation
1) 320 cm to m
2) 5100 km to m
3) 0.51 cm to m
- Continue to part 1: Scalars and Vectors
- Go to the 1D Motion Unit Page
- Back to the Stickman Physics Home Page
- Equation Sheet