Solution 1: Scalar Vector
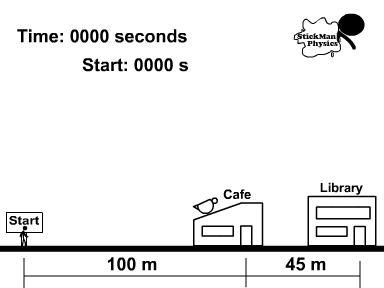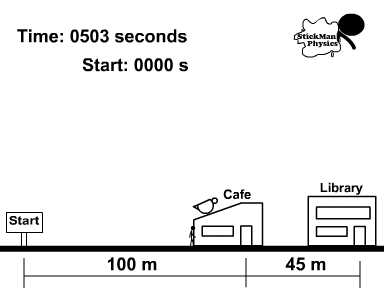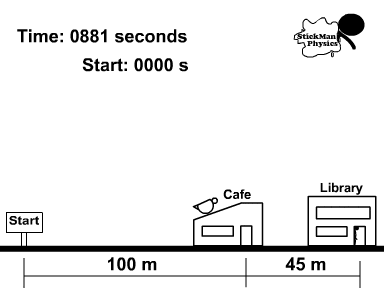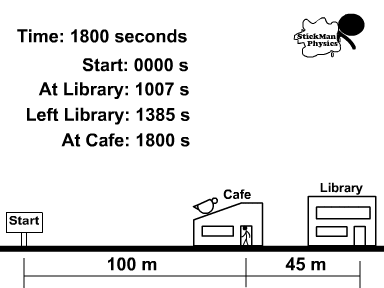
Use the picture to the right for the following questions. Thomas, on his lunch break, took 30 minutes to go to the library and then the cafe.
A. What distance did Thomas travel during lunch?
Distance is a sum of the entire measurement traveled here.
100 to the cafe then 45 more to the library then 45 back to the cafe. So add all of these up.
100 + 45 + 45 = 190 meters
B. What was his displacement?
Displacement is how far Thomas is from the origin (start) stated with direction from the origin to end point.
(100 m right) + (45 m right) + (45 m left)
Because we can't deal with directions in a calculator right becomes (+) and left becomes (-)
(+100) + (+45) + (-45) = +100
Finally we add the unit and turn the sign back (here +) into a direction.
+100 = 100 m right
C. Determine the speed of Thomas during the 30 minute (1800 second) lunch?
distance = 190 m
time = 1800 s
speed = ?
Use distance to determine speed in the equation
speed = distance/time
speed = 190/1800
speed = 0.1056 m/s
D. What was the velocity of Thomas during the 30 minutes?
displacement = 100 m right
time = 1800 s
velocity = ?
Use displacement to determine velocity in the equation
velocity = displacement/time
velocity = 100/1800
velocity = 0.0556 m/s right
(If Thomas traveled 0.0556 m/s right the entire time from the origin instead he'd end in the same place.)
Observe the animations in the scalar vector lesson if you are still having trouble with distance displacement speed and distance.