Weight, Normal Force, and Tension (Multiple Block Interactions)

Interactions with Weight, Normal Force, and Tension (Multiple Blocks)

Follow equilibrium situations that have multiple box Interaction. Create equilibrium equations to solve problems on a surface or hanging.

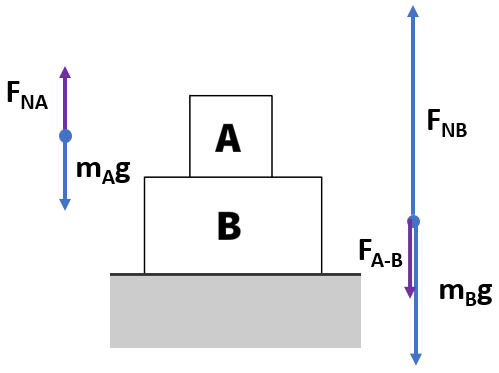
Box A on top of box B on the ground in equilibrium

What are the common force magnitudes in this scenario:

  • The weight mAg is a field force on A but equal in magnitude to the contact force (FA-B) which is the push of A down on box B.

All boxes are not moving in equilibrium (ΣF = 0 N in equilibrium)

 

Two boxes A and B stacked free body diagram

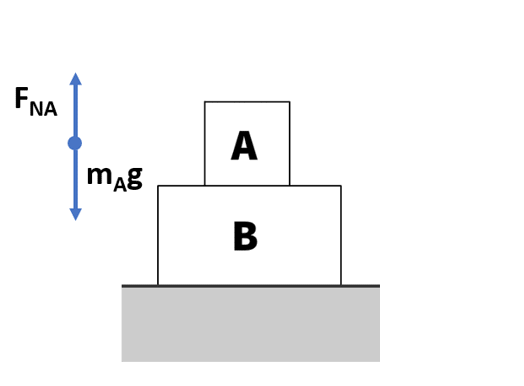
Forces on box A with up being positive:

  • Fg: -mAg
  • +FNA

ΣF = FNA – mAg

Forces on A in Box AB Situation
  • ΣF = 0 N in equilibrium

0 = FNA – mAg

FNA  = mAg

This derivation shows that the normal force which is equal to the force B pushes on A (FB-A) is equal to the weight of Box A.

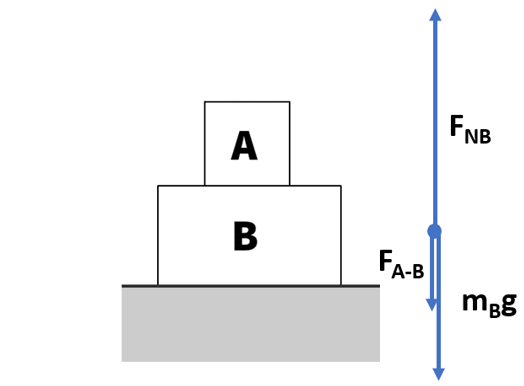
Forces on box B with up being positive:

  • Fg: -mBg
  • FA-B: -mAg
  • +FNB

ΣF = FN – FA-B – mBg

Forces on B in Box AB Situation
  • ΣF = 0 N in equilibrium

0 = FNB – FA-B – mBg

FA-B = FNB – mBg

This derivation shows that the force of A pushing on B is equal to the the normal force pushing up on B minus the weight of box B.

FNB = FA-B + mBg

This derivation shows that the normal force pushing up on B is equal to the force A pushes down on B plus the weight of box B.

Further substitution since magnitude of FA-B = mAg

ΣF = FN – mAg – mBg

  • ΣF = 0 N in equilibrium

0 = FNB – mAg – mBg

FNB = mAg + mBg

This derivation shows that the normal force pushing up on B is equal to the weight of both masses combined.

Two Boxes Hanging and Tension

What are the common force magnitudes in this scenario:

  • B is hanging with a tension up of (FT B-A) which is equal to box B’s pull down on A.
  • Box A is pulling B up by (FT A-B) which is equal and opposite to (FT B-A).

All boxes are in equilibrium (ΣF = 0N in equilibrium)

Two Hanging Boxes Tension

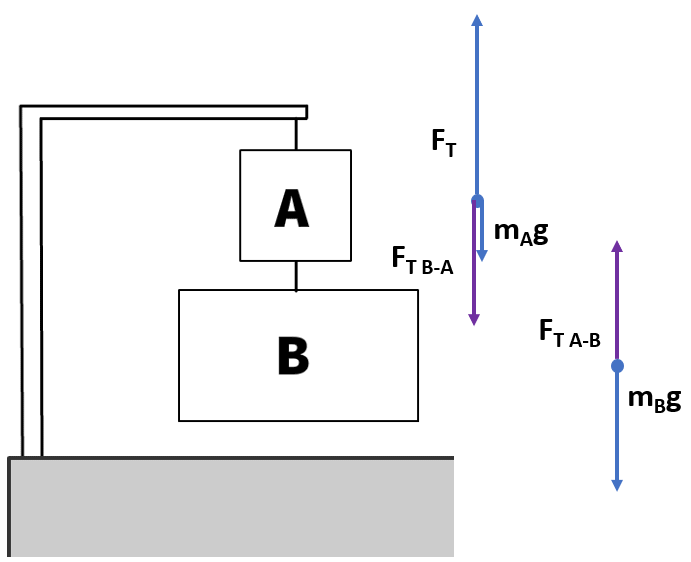
Forces on box A with up being positive:

  • Fg: -mAg
  • -FT B-A
  • +FT

ΣF = FT – mAg – FT B-A

Forces on Hanging Box A
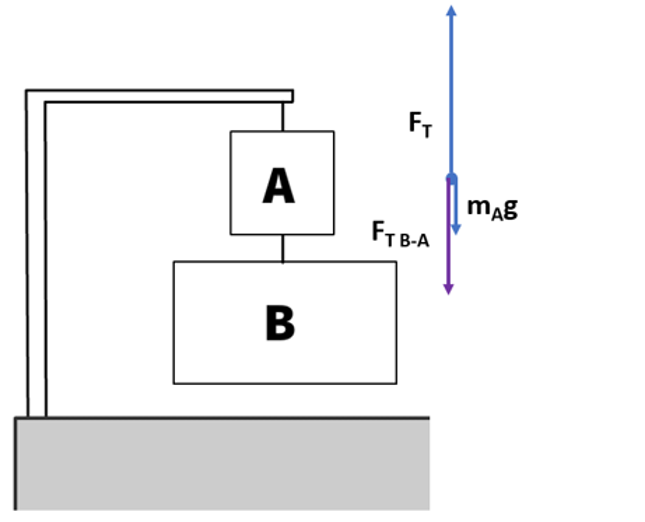
  • ΣF = 0N in equilibrium

0 = FT – mAg - FT B-A

FT = mAg + FT B-A

The tension in the rope above box A is equal in magnitude to the weight of box A plus the tension of box B pulling A down

FT B-A = FT – mAg

The tension in the rope below A is equal to the tension in the rope above A minus the weight of box A.

Forces on box B with up being positive:

  • Fg: -mBg
  • FT A-B

ΣF = FT A-B – mBg

Forces on hanging box B
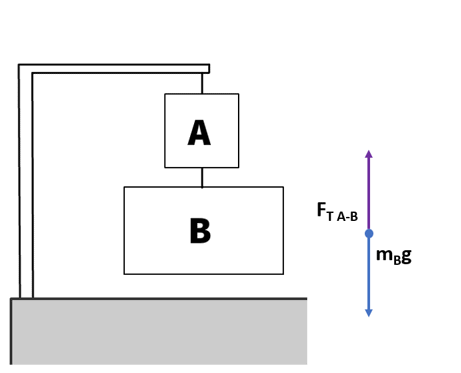
  • ΣF = 0N in equilibrium

0 = FT A-B – mBg

FT A-B = mBg

The tension in the rope above box B is equal to the magnitude of box B's weight.

Further substitution

  • FT B-A = FT – mAg
  • FT A-B = mBg

Since FT B-A = FT A-B = mBg

mBg = FT – mAg

FT = mAg + mBg

The tension in the rope above box A is equal to the weight of box A and box B combined.

Two Boxes Hanging with Tension and Normal Force

Box A hanging with box B hanging below on the ground in equilibrium

What are the common force magnitudes in this scenario:

  • B is hanging with a tension up of (FT B-A) which is equal to box B’s pull down on A.
  • Box A is pulling B up by (FT A-B) which is equal and opposite to (FT B-A).
    • This is less than the previous scenario because of the ground applying some force up on block B lessening the amount of tension in the rope between block A and B.

***note: my FT A-B subscript represents the force of tension from the block A side of the rope on block B.

Two Hanging Boxes Tension and Normal Force

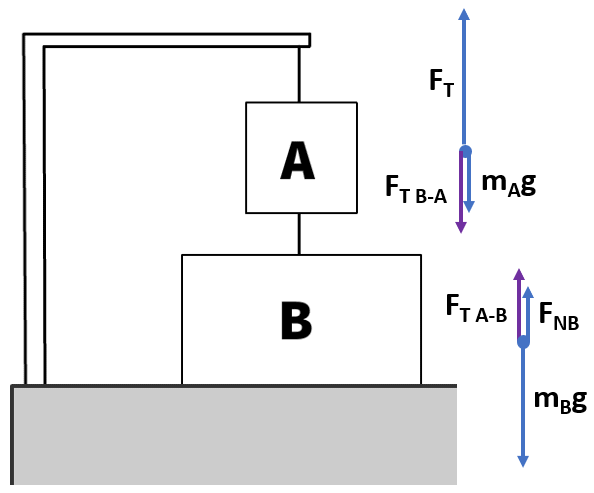
Forces on box A with up being positive:

  • Fg: -mAg
  • -FT B-A
  • +FT

ΣF = FT – mAg – FT B-A

Box A with Box B on the ground

This scenario is the same as the prior but there will be less pull down as a result of box B on the ground with normal force.

  • ΣF = 0N in equilibrium

0 = FT – mAg - FT B-A

FT = mAg + FT B-A

The tension in the rope above box A is equal in magnitude to the weight of box A plus the tension of box B pulling A down

FT B-A = FT – mAg

The tension in the rope below A is equal to the tension in the rope above A minus the weight of box A.

Forces on box B with up being positive:

  • Fg: -mBg
  • +FT A-B
  • +FNB

ΣF = FT A-B + FNB – mBg

Box B with Box B on the ground
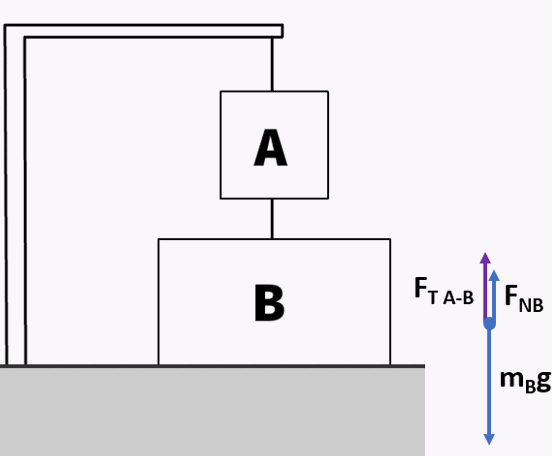
  • ΣF = 0N in equilibrium

0 = FT A-B + FNB – mBg

FT A-B = mBg - FNB

The tension in the rope above box B is equal to the magnitude of box B's weight minus the normal force of the ground pushing up.

FNB = mBg - FT A-B

The normal force of the ground pushing up is equal to the magnitude of the weight of box B minus the tension in the rope above B.

Further substitution:

  • FT B-A = FT – mAg
  • FT A-B = mBg - FNB

FT = mAg + FT B-A

The tension above box A is equal to the magnitude of box A's weight plus the tension in the rope below A.

Since FT B-A = FT A-B = mBg - FNB

FT = mAg + mBg - FNB

FT = g(mA + mB) - FNB

 

After a little more rearranging you see the tension above box A is equal to the combined weight of box A and B minus the normal force below box B.

Links

Terms and Conditions - Privacy Policy