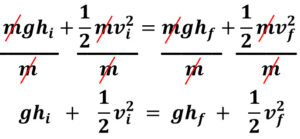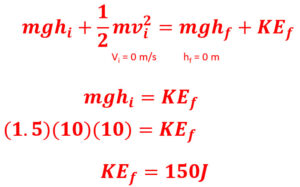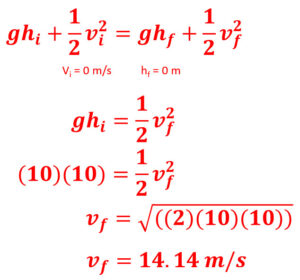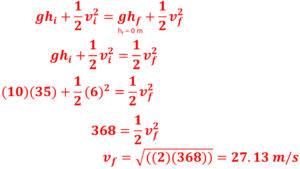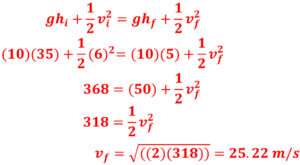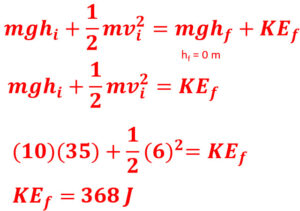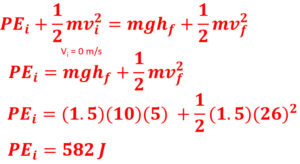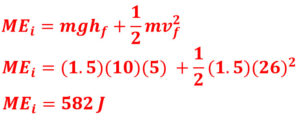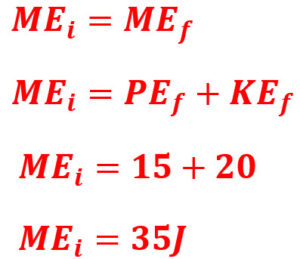Conservation of Energy
Learn about open and closed system and how to solve for problems using the conservation of energy formula with mechanical energy
Conservation of Energy Variables
| Name | Variable | Unit | Unit Abbreviation |
| Mechanical Energy | ME | Joule | J |
| Potential Energy | PE | Joule | J |
| Kinetic Energy | KE | Joule | J |
| Mass | m | Kilogram | kg |
| Acceleration Due To Gravity | g | Meters per second squared | m/s2 |
| Velocity | v | Meters per Second | m/s |
| Height or distance | h or d | Meters | m |
| Weight | Fw | Newtons | N |
Systems and Environment
A system is an object or collection of objects defined as those being studied.
- An example of a system could be a roller coaster. A smaller example may be the roller coaster cart itself
- Objects outside a system are considered external and in its environment or surroundings.
If your system was just the roller coaster cart would lose heat resulting from friction with the track. The track would be considered outside the system and in the roller coasters surroundings
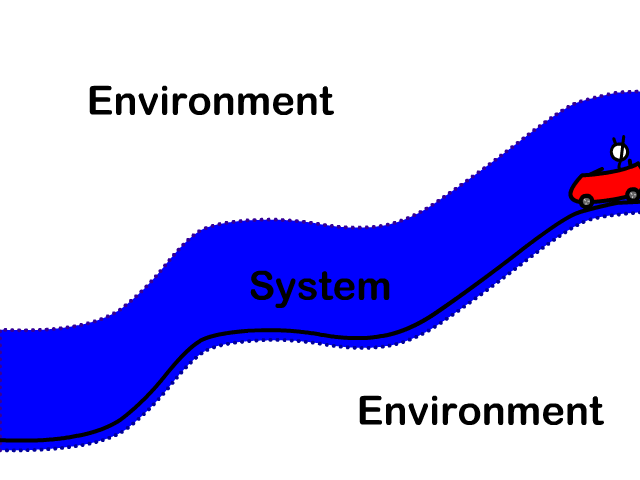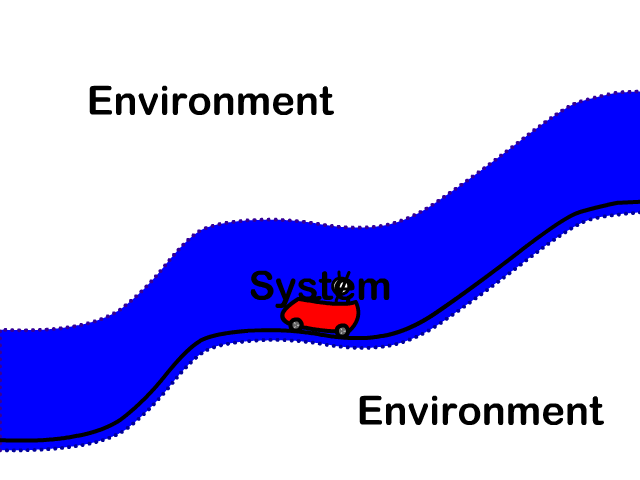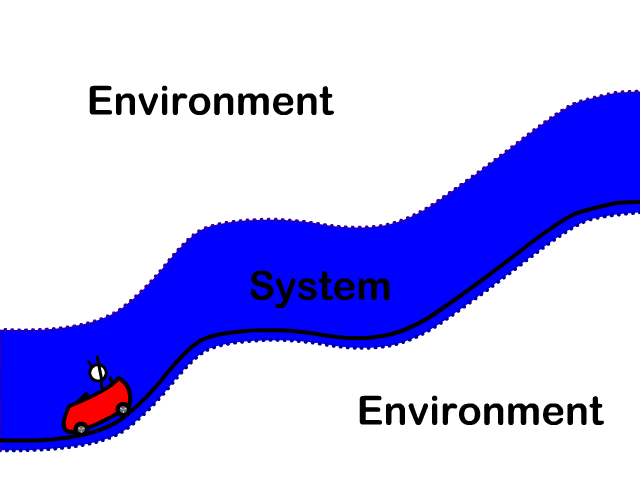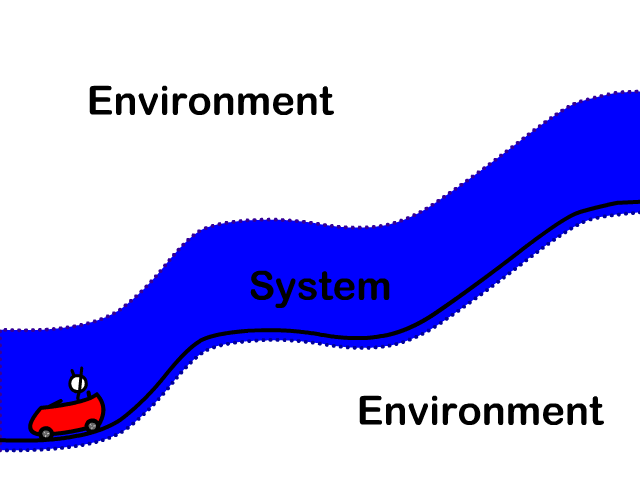
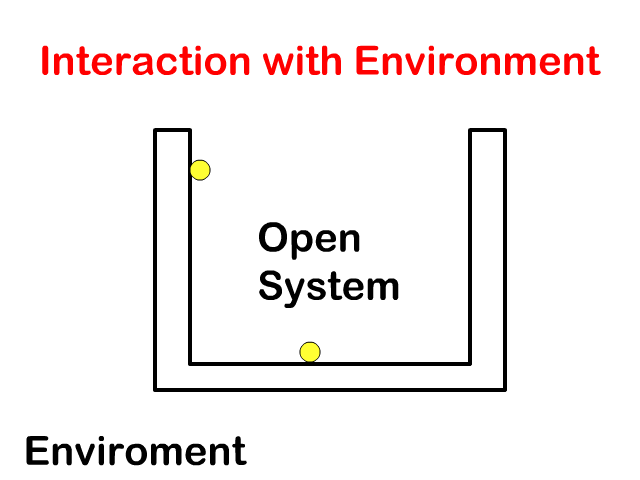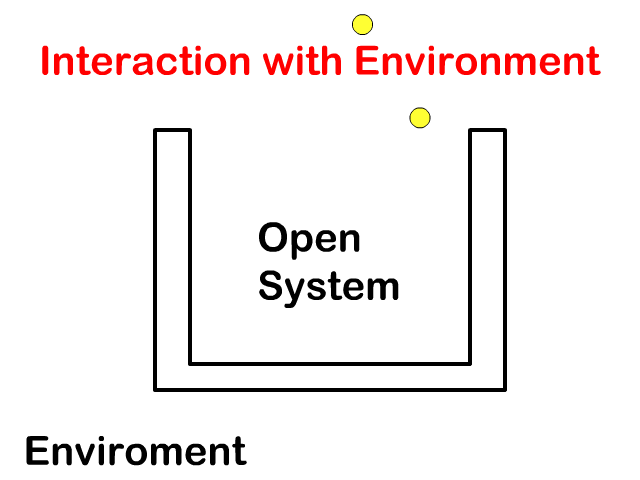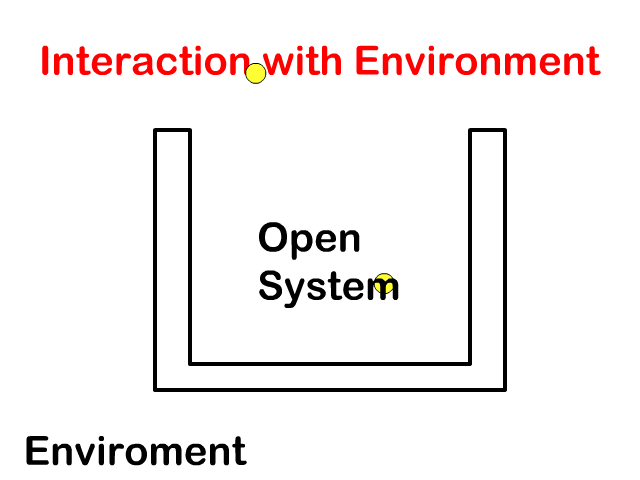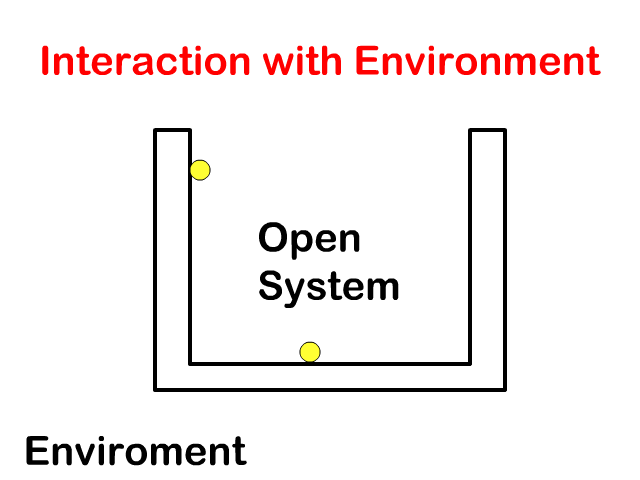
Two types of systems are open and closed systems
- A closed system does not interact with the environment and has no outside forces or energy transfer.
- An open system does interact with the environment and has external forces or energy transfer.
The Law of Conservation of Energy
Energy is neither created nor destroyed just transformed from one form to another.
- In closed or isolated systems, energy would remain constant in the system
- Open systems would have energy lost to the environment, but not lost to the universe
Different Forms of Energy
- Chemical Potential Energy
- Mechanical (ME): Kinetic Energy (KE) & Potential Energy (PE)
- Light
- Heat
- Nuclear
- Electric
When a firecracker explodes, chemical potential energy converts into light, heat, and kinetic energy.
Energy is not lost, just converted
Energy is conserved in the universe, not an open system
- A roller coaster cart would lose energy to the environment
- As potential energy converts to kinetic energy, some energy is lost as heat
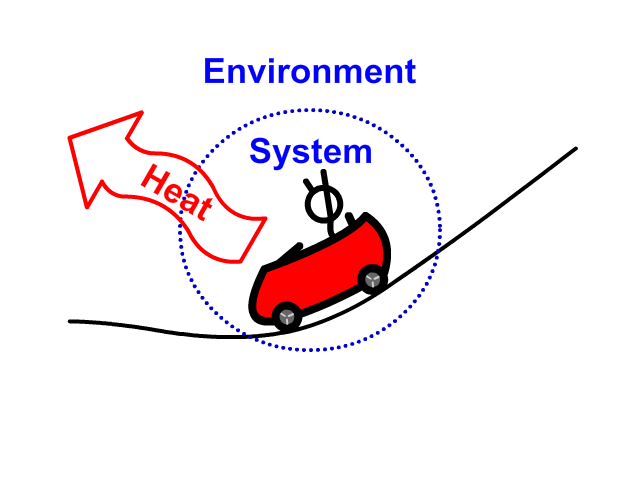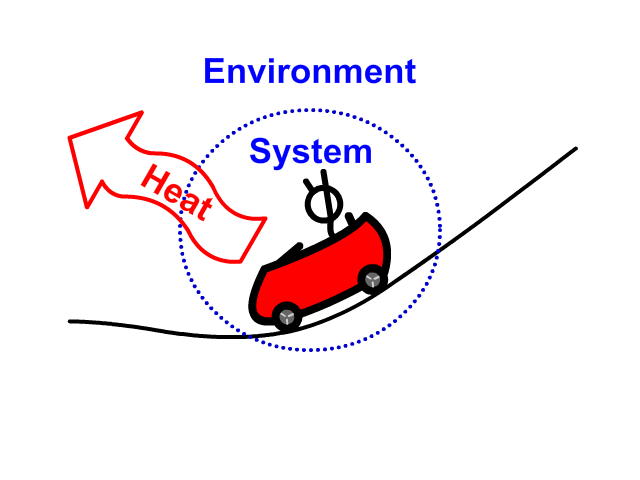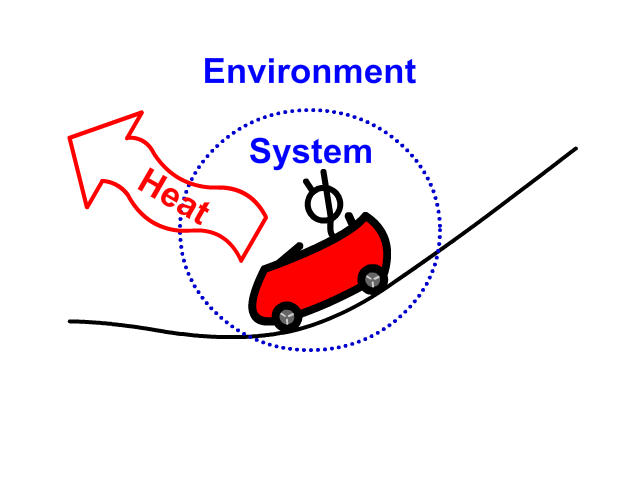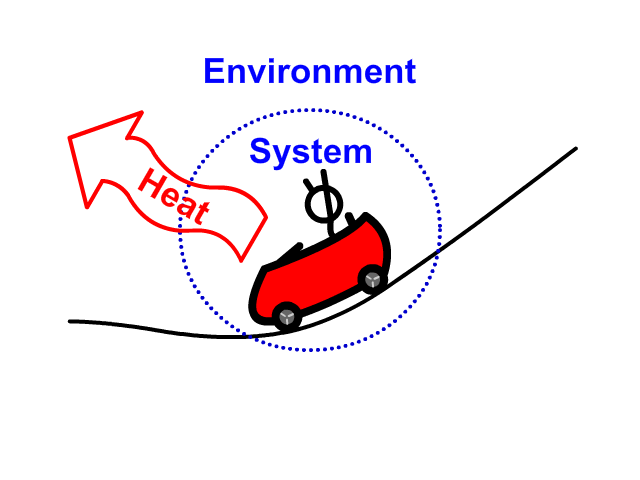
Mechanical energy is energy in motion (kinetic energy) or energy that can become motion (potential energy).
- Total mechanical energy is the kinetic energy plus potential energy.
- Our focus will be on mechanical energy and conversion of one form (potential energy) to another form (kinetic energy) in this lesson
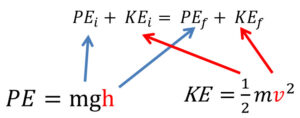
Conservation of Energy Equation
The following equations will require substitutions determined by the question itself. These substitutions will involve PE = mgh and KE = 1/2 mv² from our mechanical energy lesson found here.
Watch our video at the top of this page and walk through how to do this with us as we visit the following problems.
Mass can cancel our of the conservation of energy equation in its expanded form when each part has the mass variable.
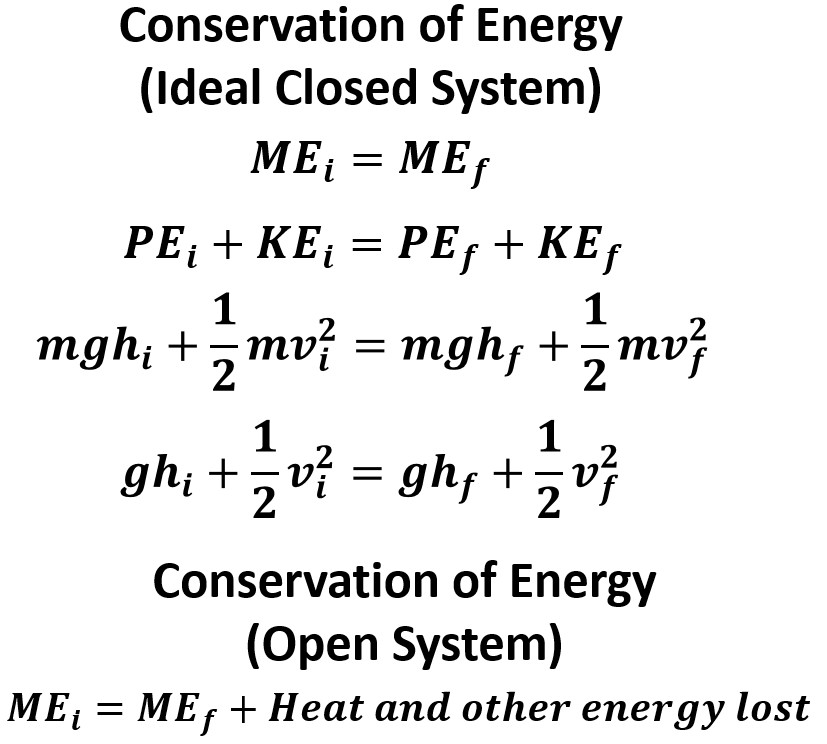
Ideal (Perfect, No Energy Lost) Situations
- Potential energy gets transformed to kinetic as an object falls
- The overall mechanical energy did not change
Example Conservation of Energy Problems
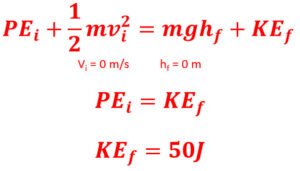
Q1: A 1.5kg rock has 50 J of potential energy when dropped. What is its kinetic energy when it hits the ground?
Q2: A 1.5kg rock is dropped from a height of 10 meters. What is its kinetic energy when it hits the ground?
Q3: A 1.5kg rock is dropped from a height of 10 meters. What is its velocity when it hits the ground?
Q4: A 1.5kg rock falling at 6 m/s is 35 m off the ground. What is its velocity when it hits the ground?
Q5: A 1.5kg rock falling at 6 m/s is 35 m off the ground. What is its velocity 5 meters off the ground?
Q6: A 1.5kg rock falling at 6 m/s is 35 m off the ground. How much kinetic energy does it have at the moment it hits the ground?
Q7: How much potential energy does a 1.5kg rock have when dropped if it is going 26 m/s when 5 meters from the ground?
Q8: How much mechanical energy does a 1.5kg rock have when dropped if it is going 26 m/s when 5 meters from the ground?
Q9: How much mechanical energy does a 1.5kg rock have when dropped has 20 J of kinetic energy and 15 J of potential energy a few seconds later?
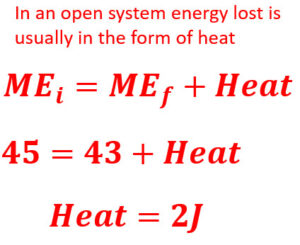
Use this equation if a problem asks for how much heat is lost:
MEi = MEf + Heat
Heat would leave an open system
Q10: If a 1.5kg rock when dropped had 45J of potential energy when dropped and 43J of kinetic energy when it hit the ground, how much converted to heat?
Conservation of Energy Problem Set
Clicking on check answer will give you the answer watch the end of the video at the top of the page to see the work to get the solution.
When you drop an object does the following increase, decrease, or stay the same in an ideal situation as it falls to the ground?
1A) Kinetic Energy
1B) Potential Energy
1C) Mechanical Energy
1D) If the situation was not ideal, what most likely would the energy be lost as?
2. How much kinetic energy does a 1.2 kg ball have the moment it hits the ground 3.5 meters below when it starts from rest?
3. How fast is a 1.2 kg ball traveling the moment it hits the ground 3.5 meters below when it starts from rest?
4. A 3.5 kg ball falls from a height of 12 meters. How fast is it traveling when still 5 meters off the ground?
5. A 3.5 kg ball falls from a height of 12 meters. How much kinetic energy does it have when still 5 meters off the ground?
6. An roller coaster cart is traveling 4 m/s at the top of a hill 61 meters off the ground. How fast is it traveling at top of a second hill 24 meters off the ground?
7. A 0.16 kg pool ball is shot off a 0.73 m high table and the ball hits the floor with a speed of 7.1 m/s. How fast was the ball moving when it left the pool table?
8A) A 0.16 kg pool ball was traveling at 12 m/s while 0.78 meters off the ground. How much mechanical energy does it have when it hits the ground?
8B) How much did it start with?
Mechanical Energy and Conservation of Energy Practice Quiz
Links
- Continue to the Next Section: Simple Machines
- Back to the Main Work, Power, Mechanical Energy, and Simple Machines Page
- Back to the Stickman Physics Home Page
- Equation Sheet