A Brief History of Roller Coasters
Roller coasters are not just rides; instead, they’re a journey through history, science, and thrill. To begin with, the physics of roller coasters has evolved significantly since their origins in 17th-century Russia. Back then, “Russian Mountains”—giant wooden ramps covered in ice—were constructed purely for entertainment. Furthermore, riders would slide down these icy slopes at speeds of up to 40 mph, demonstrating that humans have always craved excitement. (Learn more about the history of roller coasters)
In the early 19th century, the French adapted this concept with rides like Les Montagnes Russes à Belleville (“The Russian Mountains of Belleville”) in Paris, which featured wheeled carts locked to wooden tracks. These advancements set the stage for modern roller coasters.
The first roller coaster resembling today’s designs debuted in 1884 at Coney Island, New York. Called the Switchback Railway, it reached just 6 mph—a far cry from the high-speed rides we enjoy today—but it was a groundbreaking moment for amusement park history. (Read about early roller coaster innovations)
The Physics Behind the Thrills
Mechanical Energy: The Driving Force of the Ride
Roller coasters operate primarily on mechanical energy, which is the sum of two key energy types:
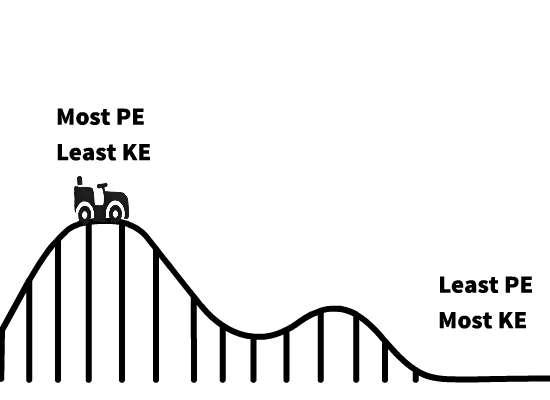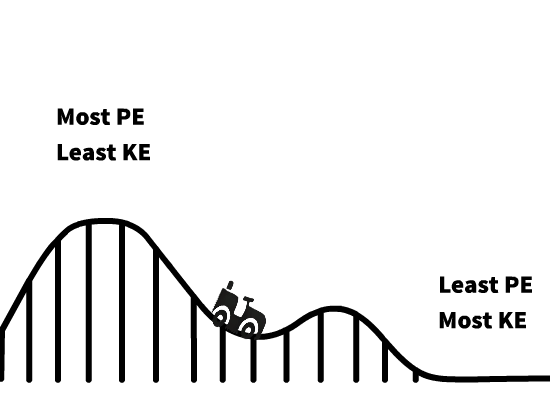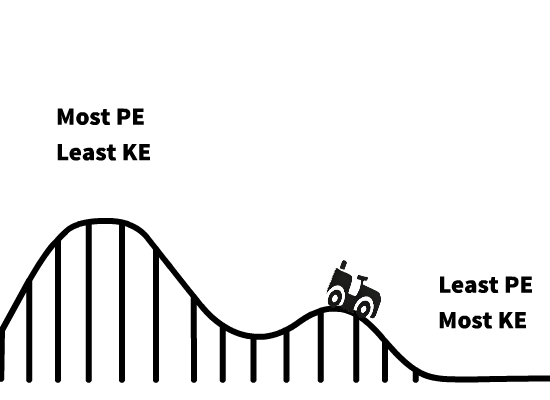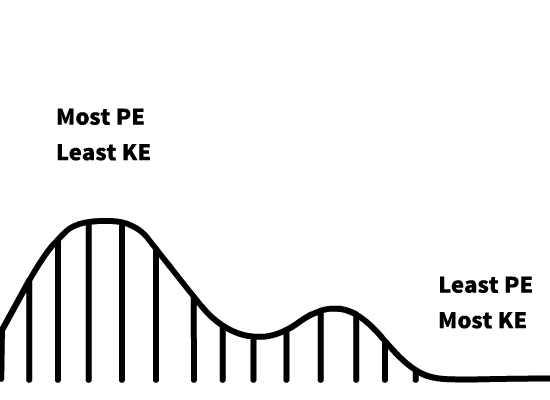
1. Potential Energy (PE):
Stored energy based on height, potential energy is at its maximum when the coaster is at the peak of a hill. The formula is:
PE = mgh
Where: PE = Potential Energy, m = mass of the coaster, g = acceleration due to gravity (9.8 m/s²), and h = height above the ground. (Learn about potential energy and see example calculations)
2. Kinetic Energy (KE):
Energy of motion, kinetic energy increases as the coaster gains speed on its descent. The formula is:
KE = ½ mv2
Where: KE = kinetic energy, m = mass of the coaster, and v = velocity of the coaster
(Explore kinetic energy and example problems)
The Law of Conservation of Energy
The law of conservation of energy states that energy cannot be created or destroyed; rather, it can only be transformed. Specifically, in mechanical systems, energy transitions between potential energy and kinetic energy both before and after an event. Consequently, the equation representing the mechanical energy during the transition is as follows:
PEi + KEi = PEf + KEf
and expanded into components as:
mghi + ½ mv i 2 = mghf + ½ mv f 2
Mechanical Energy of a Roller Coaster
On a roller coaster, at the top of the first hill, the coaster possesses maximum potential energy and minimal kinetic energy. As it begins to descend, the potential energy is gradually converted into kinetic energy, and consequently, the coaster’s speed increases. Meanwhile, friction and air resistance come into play, steadily working against the motion and converting some of the coaster’s energy into thermal energy (heat). As a result, this energy loss explains why, over time, each successive hill on the roller coaster is slightly lower than the one before it.
(Learn about the conservation of energy)
Apparent Weight: The Normal Force at Play
On a roller coaster, your apparent weight—the sensation of feeling heavier or lighter—is directly related to the normal force acting on your body. Apparent weight is the result of, and equal to, the normal force, which is the upward force exerted by the seat against gravity and any additional acceleration.
At the top of a hill, the coaster’s downward acceleration reduces the normal force, making you feel lighter and sometimes even weightless. This thrilling sensation occurs because gravity is momentarily unopposed by the seat’s upward push. On the other hand, at the bottom of a dip, the coaster’s upward acceleration increases the normal force, causing you to feel much heavier than usual. These shifts in apparent weight are what make roller coasters so exciting, as they provide a dynamic and thrilling experience at every turn.
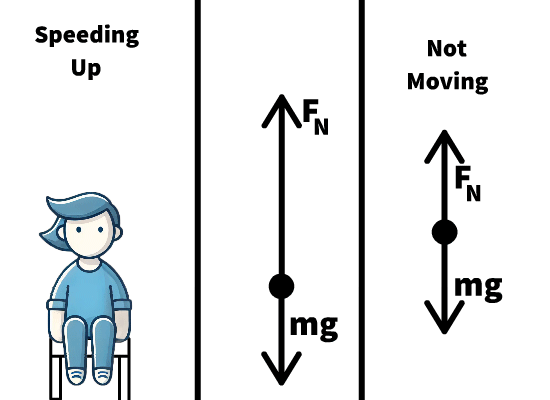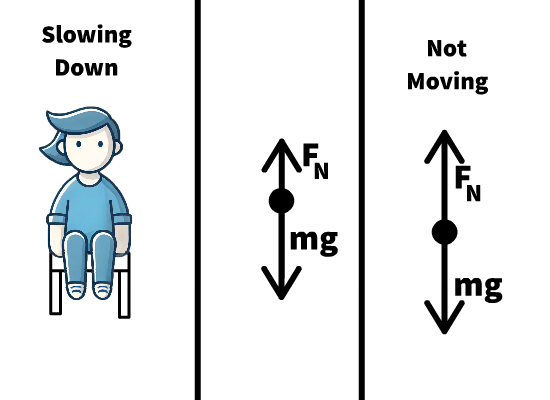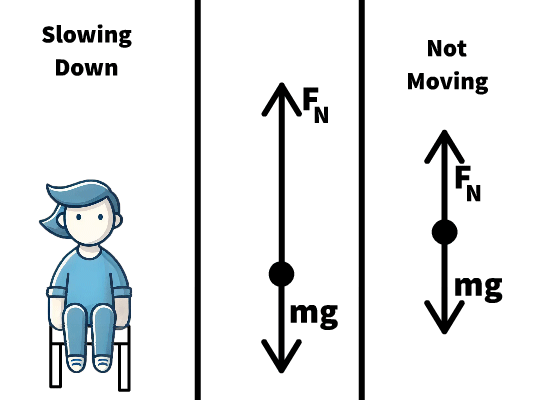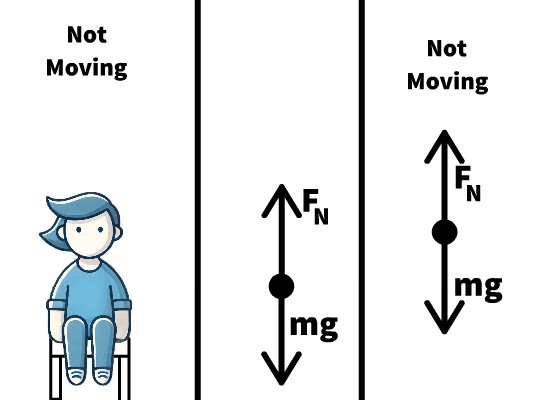
Description of the Animation: Up is Positive, Down is Negative
This animation illustrates the motion and accompanying force diagram of a person experiencing various phases of movement. Observe how the normal force and net force change throughout the animation, influencing the acceleration and velocity.
- On the Way Up, Speeding Up:
Starting from rest, the person begins moving upwards, accelerating with a positive velocity (+v) and positive acceleration (+a). The net force points upward, causing this upward acceleration. Notice that the normal force (upward) is greater than the weight (mg), creating the upward net force. - On the Way Up, Slowing Down:
Although the person is still moving upwards (+v), they are slowing to a stop with a negative acceleration (-a). This occurs because the net force has now switched to a downward direction. The normal force is now less than the weight (mg), which remains constant, leading to the downward net force. - On the Way Down, Speeding Up:
The person transitions to moving downward with a negative velocity (-v) while continuing to accelerate downward (-a). The net force remains in a downward direction unchanged. The normal force remains less than the weight (mg), sustaining the negative net force and downward acceleration. - On the Way Down, Slowing Down:
The person is still moving downward (-v), but now they are slowing to a stop with a positive acceleration (+a). This reversal happens because the net force has switched direction to point upwards. The normal force has increased and is now larger than the weight (mg), creating the upward net force necessary to bring the person to rest. - At Rest:
Finally, the person comes to a complete stop. At this point, the net force is 0 N, as the upward normal force perfectly balances the downward weight (mg). With no net force, there is no acceleration and no velocity.
This animation highlights the dynamic relationship between motion, forces, and acceleration while clearly showcasing how changes in net force impact movement direction and speed.
G-Forces and the Limits of Acceleration
Roller coasters and airplanes alike expose passengers to g-forces, which measure acceleration relative to Earth’s gravity (g=9.8 m/s2). For instance, experiencing “2 g’s” means the normal force acting on your body is twice your normal weight, making you feel significantly heavier. This effect becomes most noticeable during loops, sharp curves, or sudden dips, where acceleration changes rapidly and dramatically.
However, it’s important to note that the human body has limits when it comes to g-forces. If a coaster transitions vertically too quickly, extreme g-forces can reduce blood flow to the brain, leading to a blackout feeling. This occurs when vision dims or disappears because the brain isn’t receiving enough oxygen. For most people, brief exposure to g-forces of up to 4 to 6 g’s is tolerable. However, sustained g-forces beyond this range can cause G-LOC (G-force-induced Loss of Consciousness).
To ensure safety, roller coaster designers carefully calculate g-forces to create rides that are both thrilling and safe. By balancing speed, curvature, and acceleration, they deliver exciting experiences without pushing the limits of what humans can endure.
Loops and Turns: Centripetal Force at Work
When navigating loops and turns, riders experience multiple forces that combine to create the centripetal force, pulling the coaster inward toward the center of its circular path. As a result, this force keeps the coaster securely on the track, even when it’s upside down during a loop. Without the interaction of the weight and normal force generating a centripetal net force, the coaster would fail to maintain circular motion.
Modern roller coasters are designed with clothoid loops, which are teardrop-shaped rather than perfectly circular. This is because clothoid loops help reduce extreme g-forces at the bottom of the loop, making the ride smoother and safer for riders. At the top of a loop, centripetal force combines with gravity to reduce your apparent weight, creating a feeling of lightness. Conversely, at the bottom of the loop, centripetal force opposes gravity, making you feel significantly heavier.
Centripetal force is an essential component of roller coaster design. It allows engineers to balance the need for thrilling experiences with the safety and comfort of the riders. (Learn more about centripetal force in motion)
For Those Looking to Feel These Physics Principles Firsthand, Here Are Some Must-Ride Roller Coasters
1. Steel Vengeance at Cedar Point (Ohio):
A record-breaking hybrid coaster that seamlessly combines the best of wood and steel. Moreover, it boasts the longest airtime of any coaster and clearly demonstrates energy transformations in every hill and drop. (Explore Cedar Point)
2. Twisted Colossus at Six Flags Magic Mountain (California):
This impressive hybrid coaster not only features synchronized racing trains but also effectively showcases kinetic energy through its high-speed turns and drops. (Visit Six Flags Magic Mountain)
3. The Incredible Hulk Coaster at Universal Studios Florida:
This high-speed ride launches riders with incredible acceleration, illustrating how potential energy can be skipped entirely for kinetic thrills. (Learn more about The Hulk Coaster)
4. Space Mountain at Disney Parks:
An indoor roller coaster that takes you on a space-themed adventure. Though less intense than other rides, it showcases controlled speed and energy shifts. (Explore Space Mountain)
Physics of Roller Coasters: Why Roller Coasters Fascinate Us
Roller coasters are more than just thrilling rides; in fact, they’re a remarkable testament to human creativity and physics in action. By seamlessly combining science, engineering, and fun, they create experiences that captivate people of all ages.
Moreover, if you enjoyed learning about the physics of roller coasters, consider sharing this article with a friend or fellow thrill-seeker. The next time you find yourself at an amusement park, take a moment to truly appreciate the incredible science that powers each ride. After all, physics is what makes the world—and roller coasters—go round!

