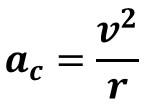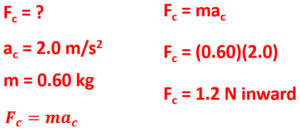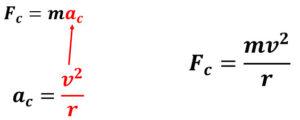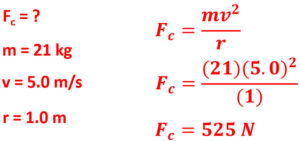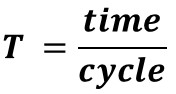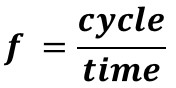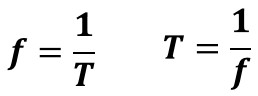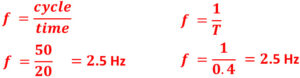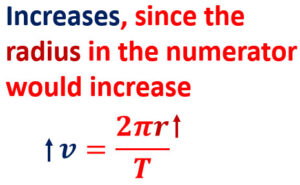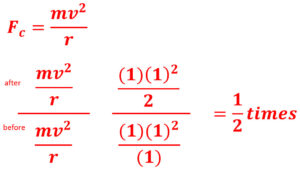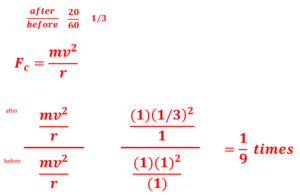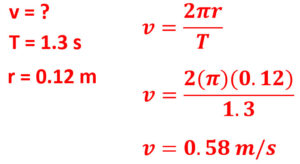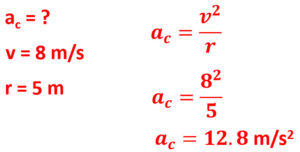Circular Motion

Home » Unit 4: Universal Gravitation and Circular Motion » Circular Motion

Circular motion requires an inward force, centripetal force, to create a change in direction resulting in a circular pattern.

Go through this lesson with our video or read and do the practice checking your work with our solutions below.

Two Types of Circular Motion

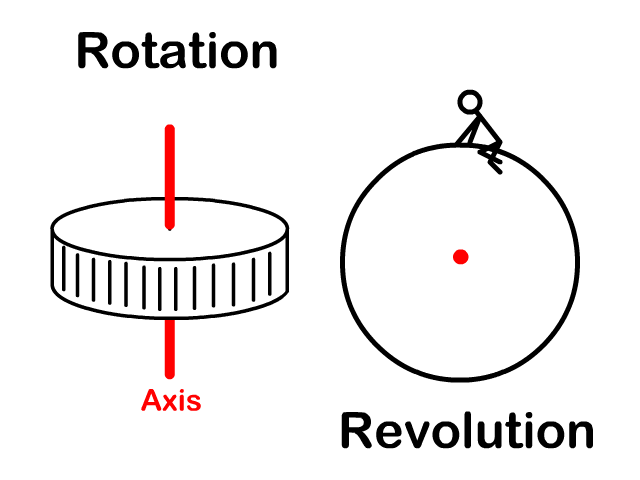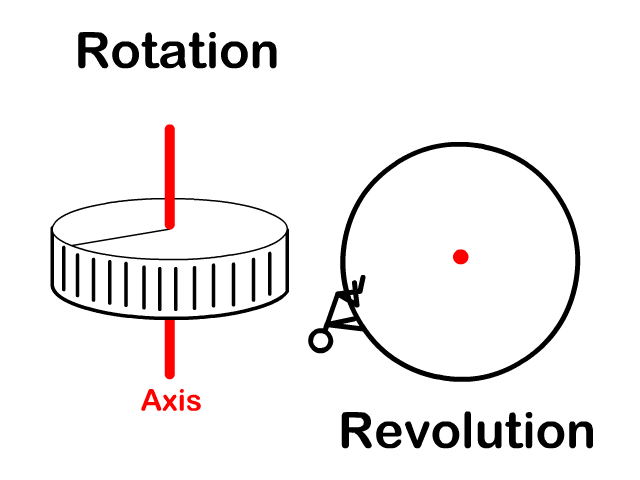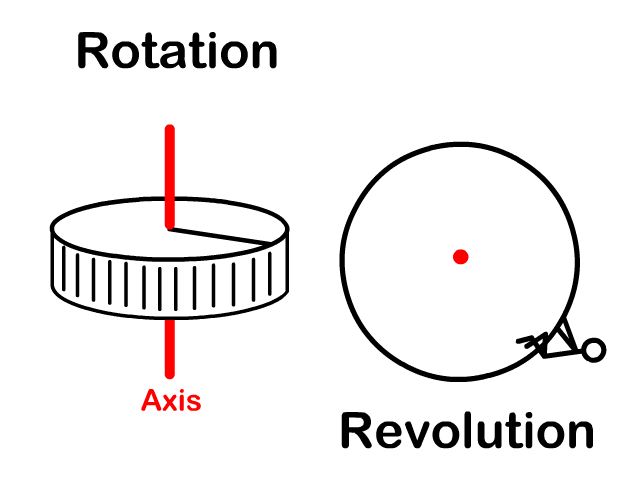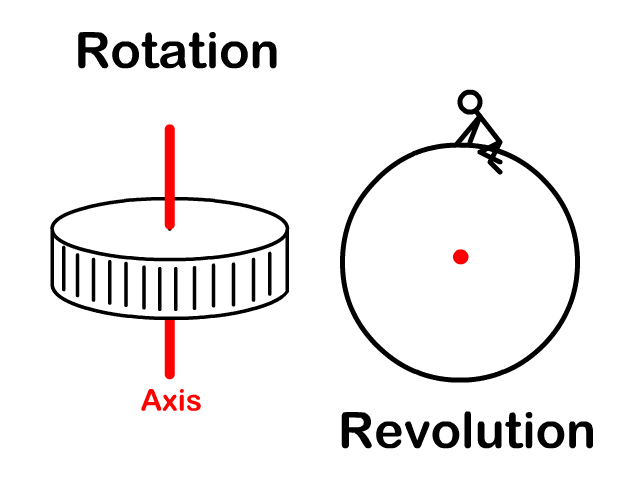
A rotation occurs when an entire object spins around an internal axis. A revolution is when an object is located beyond the axis and follows a circular path around beyond the axis. See how the stickman is revolving around the central axis. The axis is a central point around which rotation takes place.

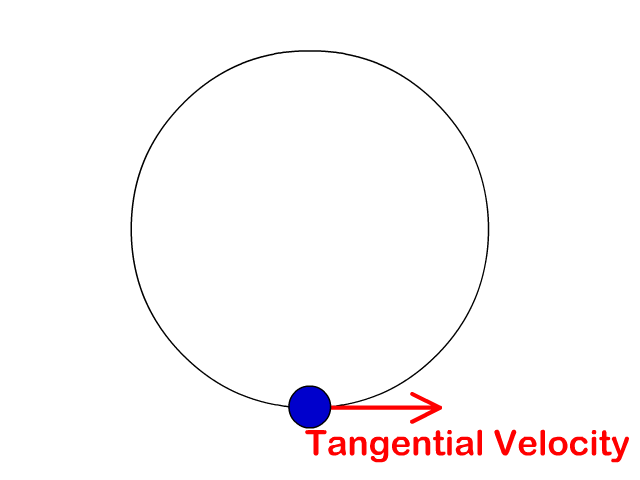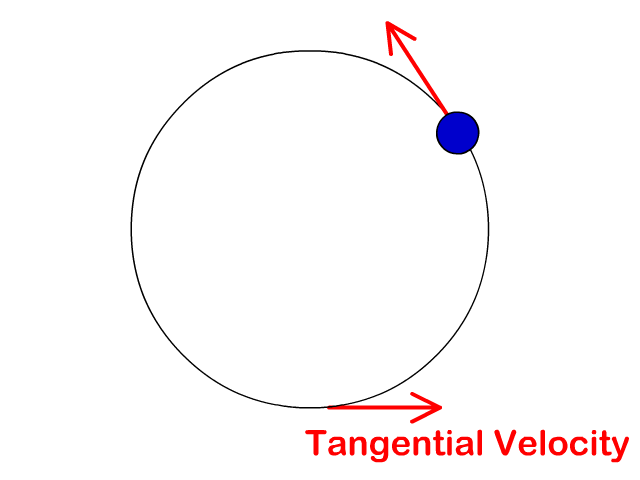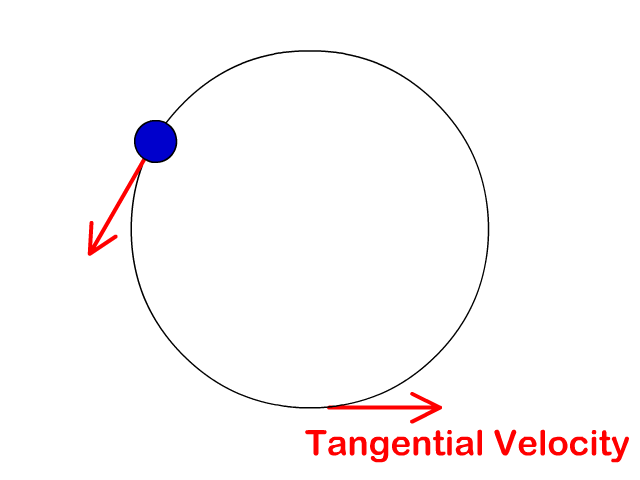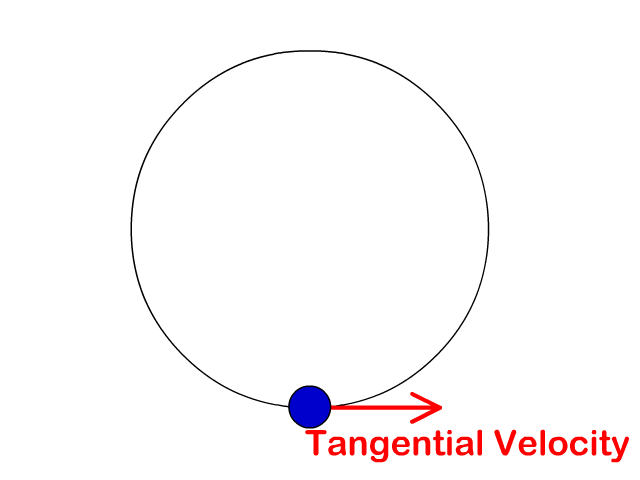
Tangential Velocity

The tangent is a straight line that would just touch the curve of the circle as seen in the animation.

Because velocity is a vector, it includes magnitude and direction. So will use the term tangential velocity to represent what the velocity would be if released and allowed to follow a straight line path.

Inertia Versus Circular Motion

Inertia makes an object want to continue forward at a constant velocity
We need a inward force to change direction and create circular motion
This inward force is centripetal force (F_c)

Centripetal Force

Centripetal Force (F_c) is an inward force towards the center of a circle, changing the direction, keeping an object in a circular path.

Since direction changes due to centripetal force, circular motion is accelerated and we call this centripetal acceleration (a_c).

If you lost the centripetal force the object would stop revolving and continue in a straight line tangent to the circumference of the circle.

Q4: What would happen to a rubber stopper in circular motion if there were no centripetal force?

It would move straight ahead tangent to the circumference of the circle

Q5: What is tangential velocity?

Velocity straight ahead from the circumference of a circle

Q6: What force is necessary to have circular motion?

Centripetal Force

Q7: What direction is this force?

Towards the center of the circle

Q8: A moving object’s inertia make it want continue ______________________________.

Straight ahead at a constant velocity

Circular Motion Variables

Variable	Name	Unit	Unit Abbreviation
F_c	centripetal force	Newton	N
m	Mass	kilogram	kg
a_c	centripetal acceleration	meters per second squared	m/s²
v or v_tangential	velocity or tangential velocity	meters per second	m/s
r	radius	meters	m
T	period	seconds	s
f	frequency	hertz	Hz

Centripetal Force Causes Centripetal Acceleration

Centripetal force creates centripetal acceleration related to mass is seen in this equation:

Centripetal acceleration is equal to the velocity squared divided by the radius seen in this equation:

Q9: How much centripetal force is required to create an inward acceleration of 2.0 m/s² to keep a 0.60 kg object in a circular path?

Q10: What is the velocity of an object going in a circle that has a radius of 1.2 meters and an inward acceleration of 4.5 m/s²?

The result after putting the previous two equations together is this main centripetal force (F_c) equation

Q11: How much centripetal force is required to cause a 21 kg object to rotate with a speed of 5.0 m/s in a circle with a radius of 1.0 meters?

Sources of Centripetal Force

What provides the centripetal force allowing for circular motion?

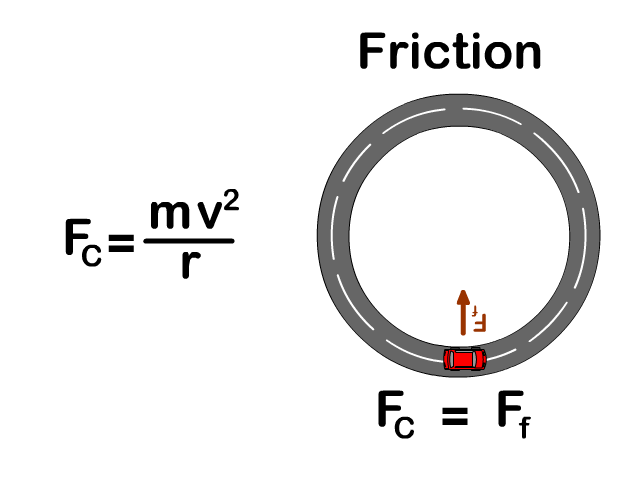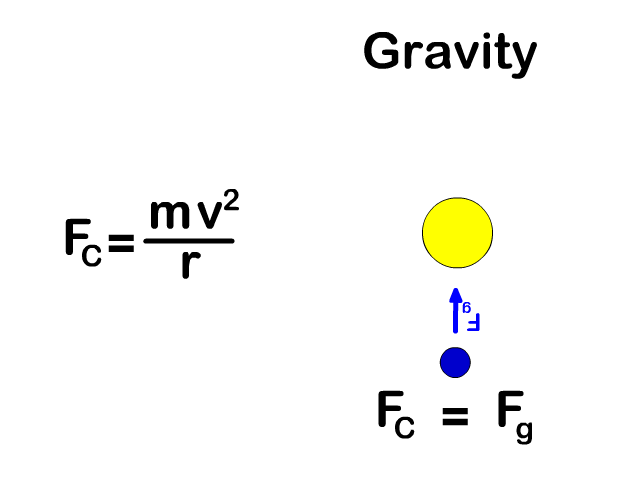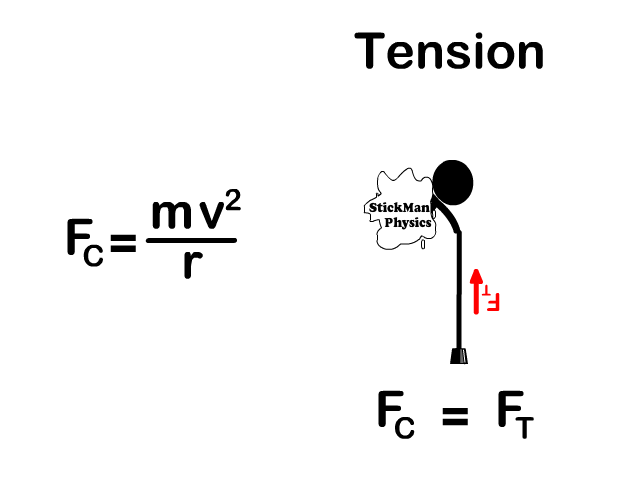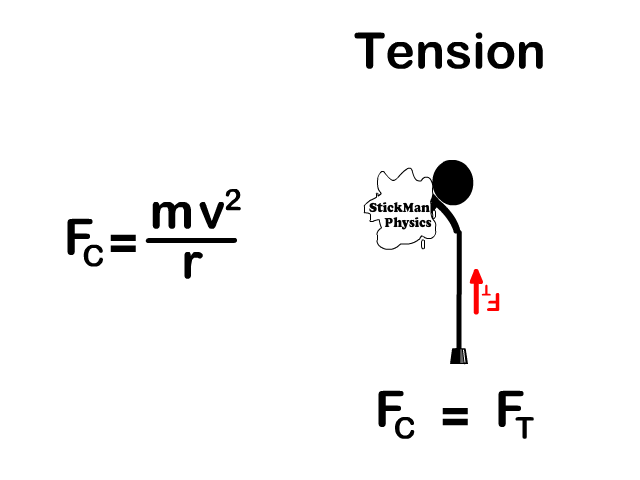
Friction for a car to drive around a corner

- - F_c = F_f friction

Gravity for the moon to go around earth

- - F_c = F_g gravity

Tension for spinning something around your head with a rope

- - F_c = F_T tension in the rope

Important Note: The problems in this unit may not state centripetal force directly. They may ask what is the tension, gravity, or friction keeping an object in circular motion. If stated this way, solve for centripetal force (F_c).

What is a Period?

Period (T) is the time it takes for a specific motion to occur. See in the animation how this could be one swing, rotation, wave, segment, or osculation. In this unit it will be a rotation.

Period and Frequency

Period (T) is the time it takes for one cycle and has the unit second (s)

Period Equation

Frequency (f) is the number of cycles per time and has the unit Hertz (Hz) which also means cycles per second

Frequency Equation:

You can find either period or frequency with number of cycles and time that those took or with inverse of frequency or period.

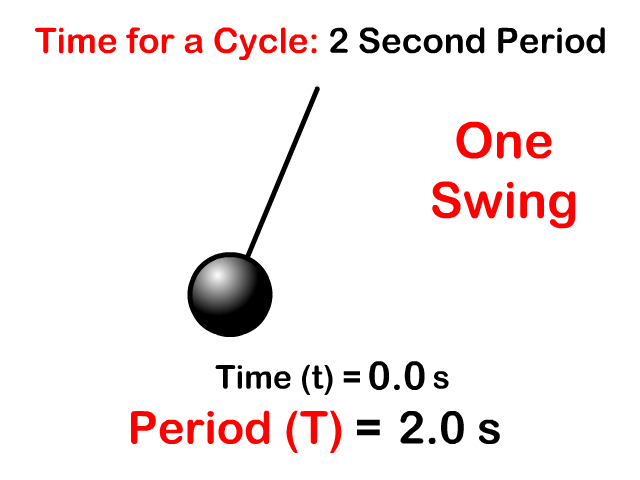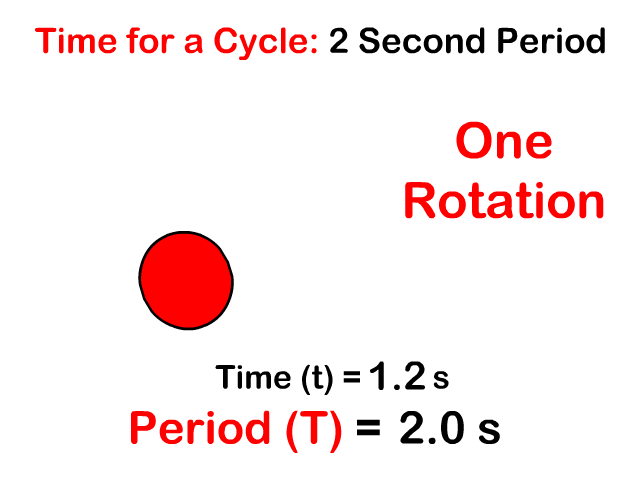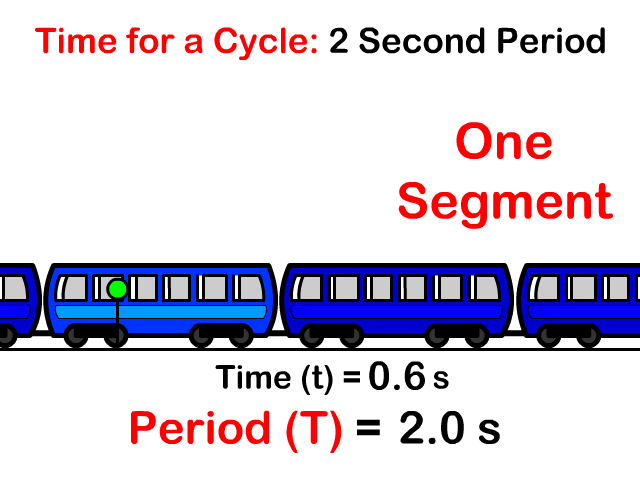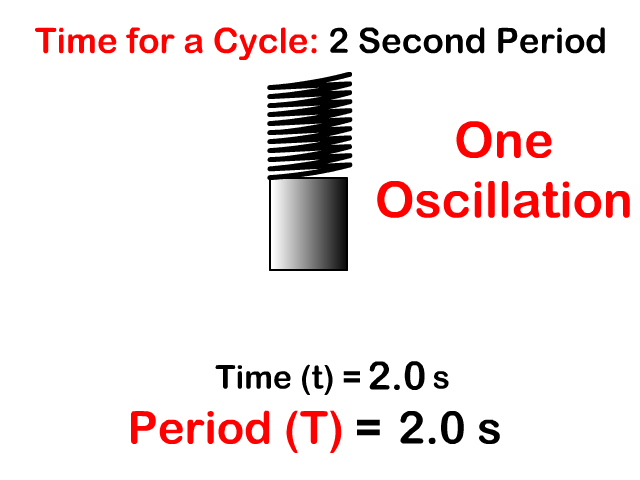
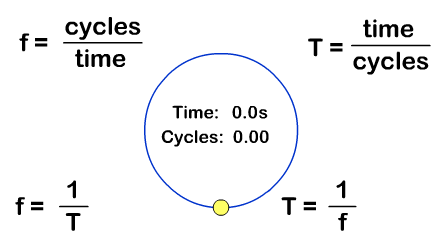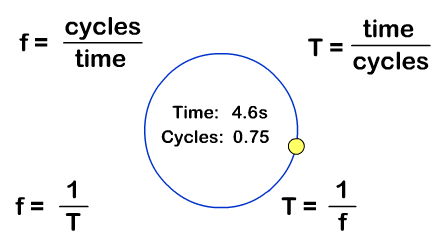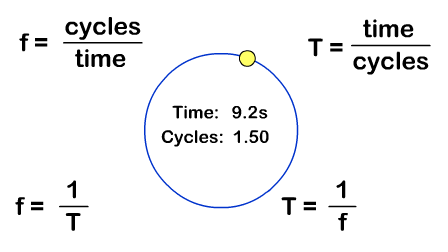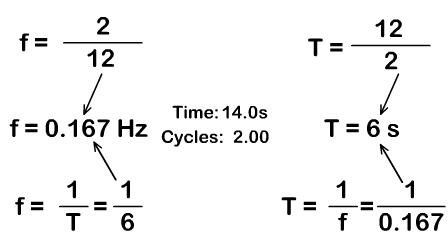
Q12: What is the period when 50 rotations occur in 20 seconds?

Q13: What is the frequency when 50 rotations occur in 20 seconds?

You can now solve this two ways after doing question 13

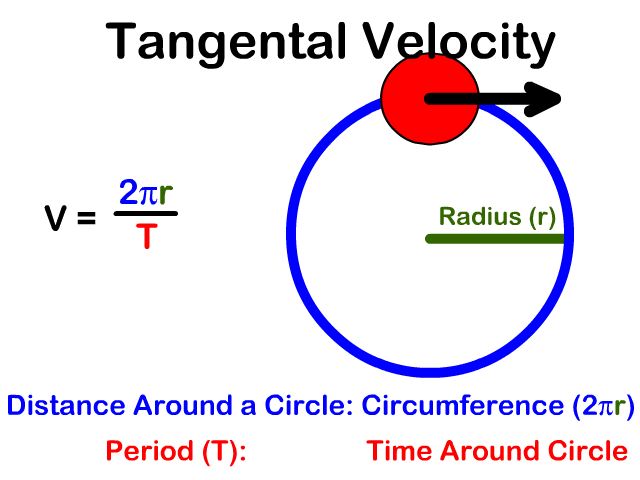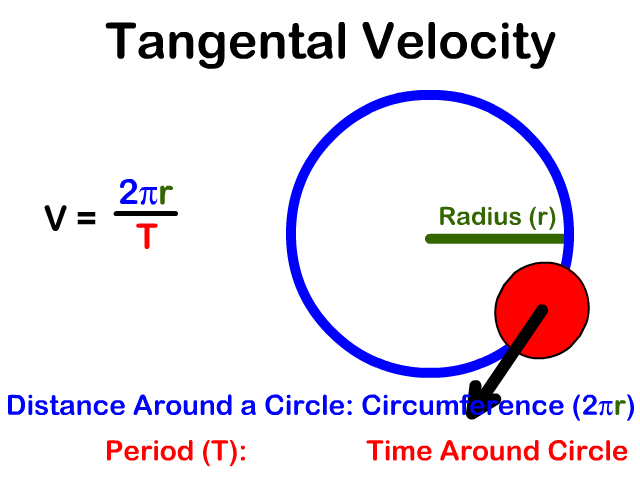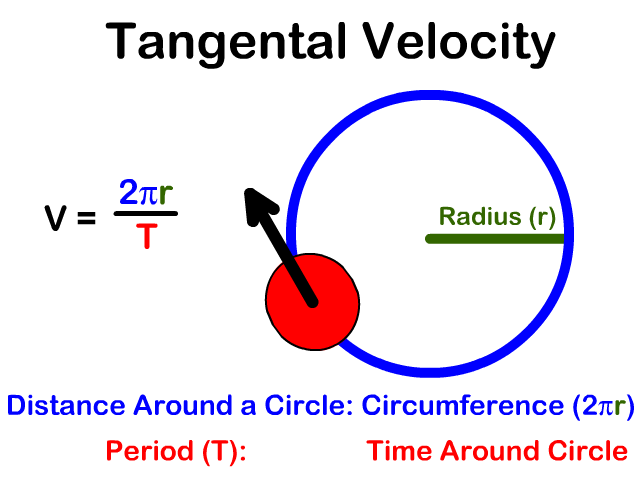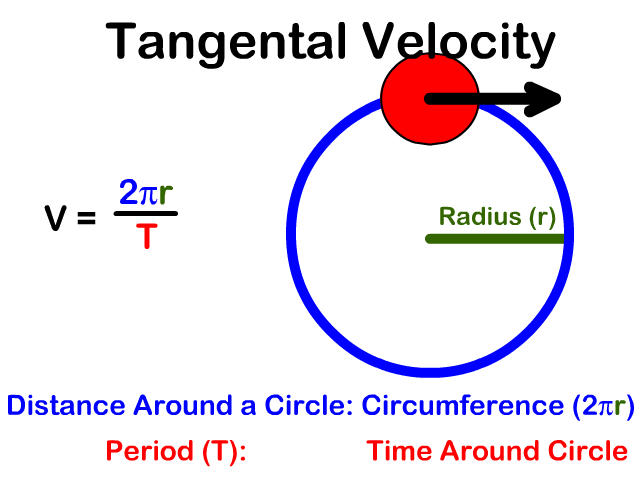
Tangential Velocity Equation

Tangential velocity is calculated by taking the circular distance divided by the time it takes to go around the circle or period (T). The distance around the circle is found by calculating the circumference of a circle.

2πr = circle circumference (unit: meters (m))

T = Period: Time it takes for one rotation (unit: seconds (s))

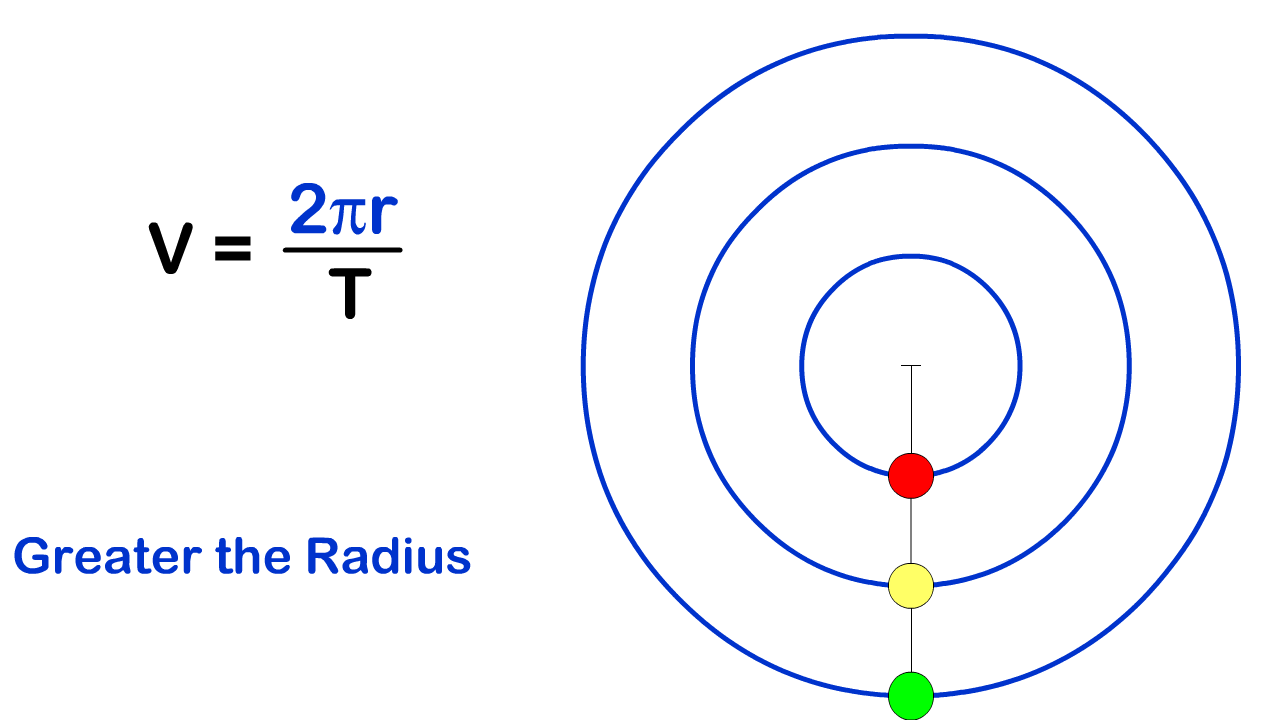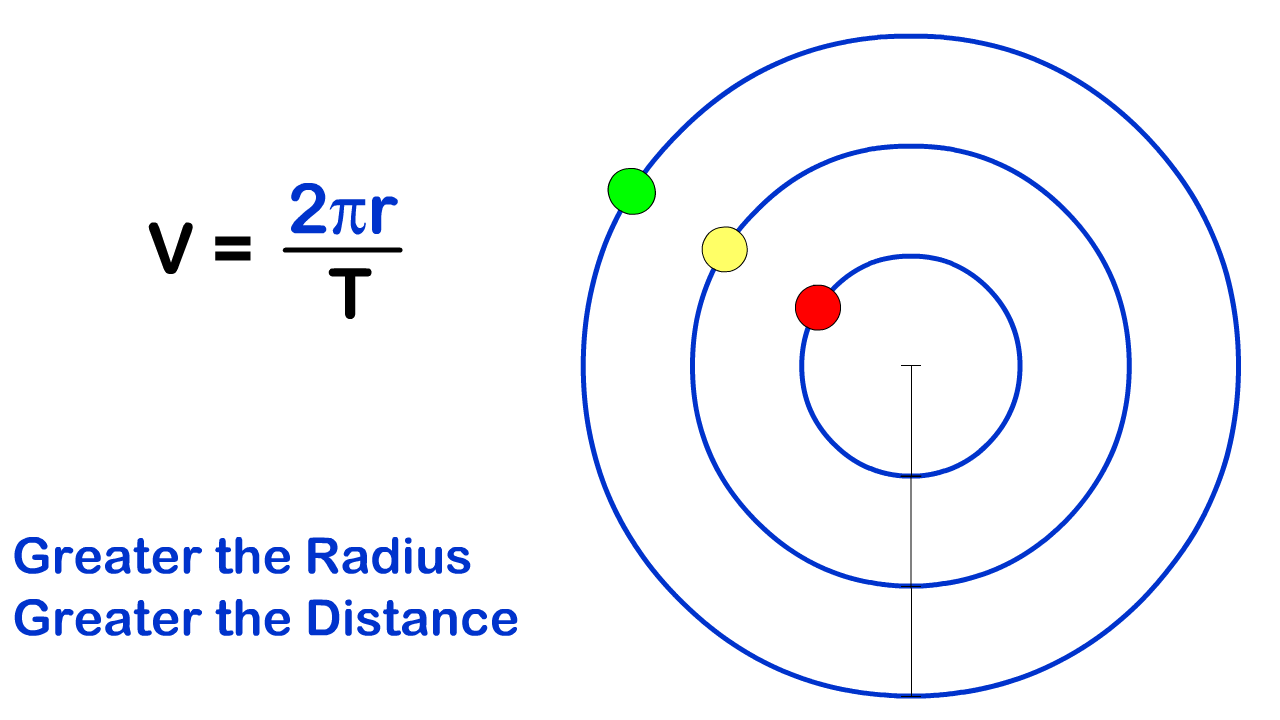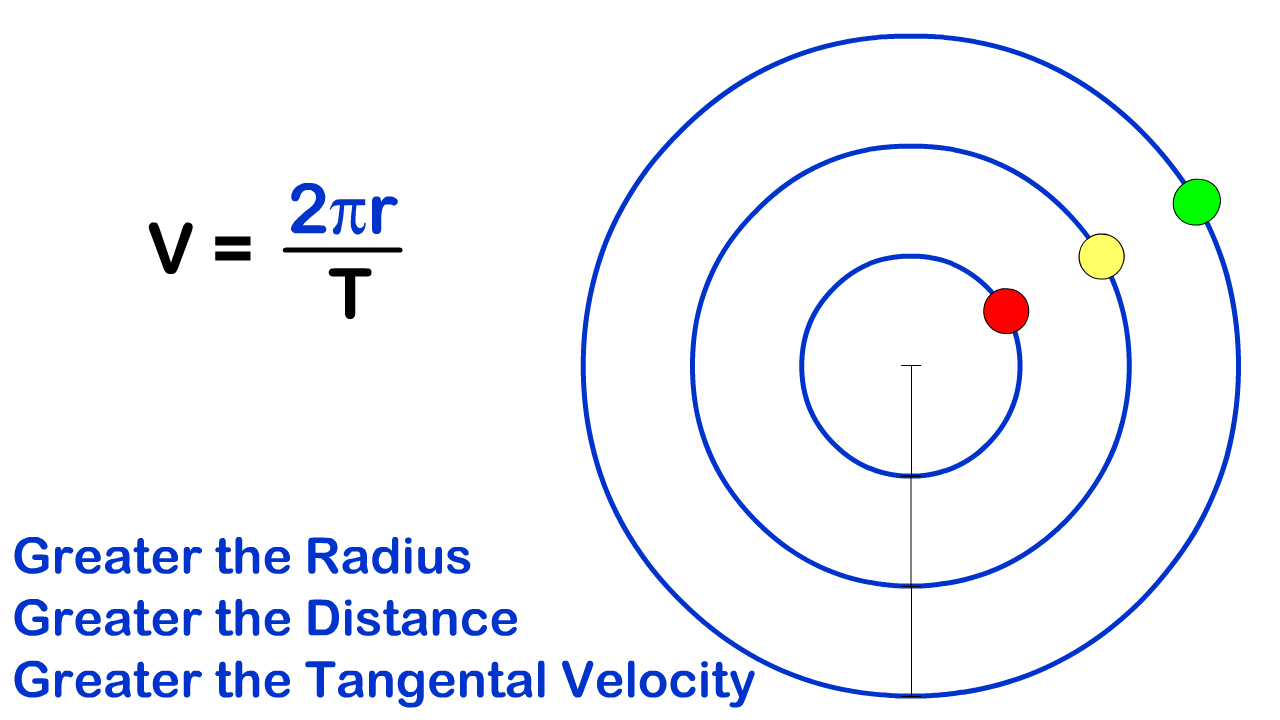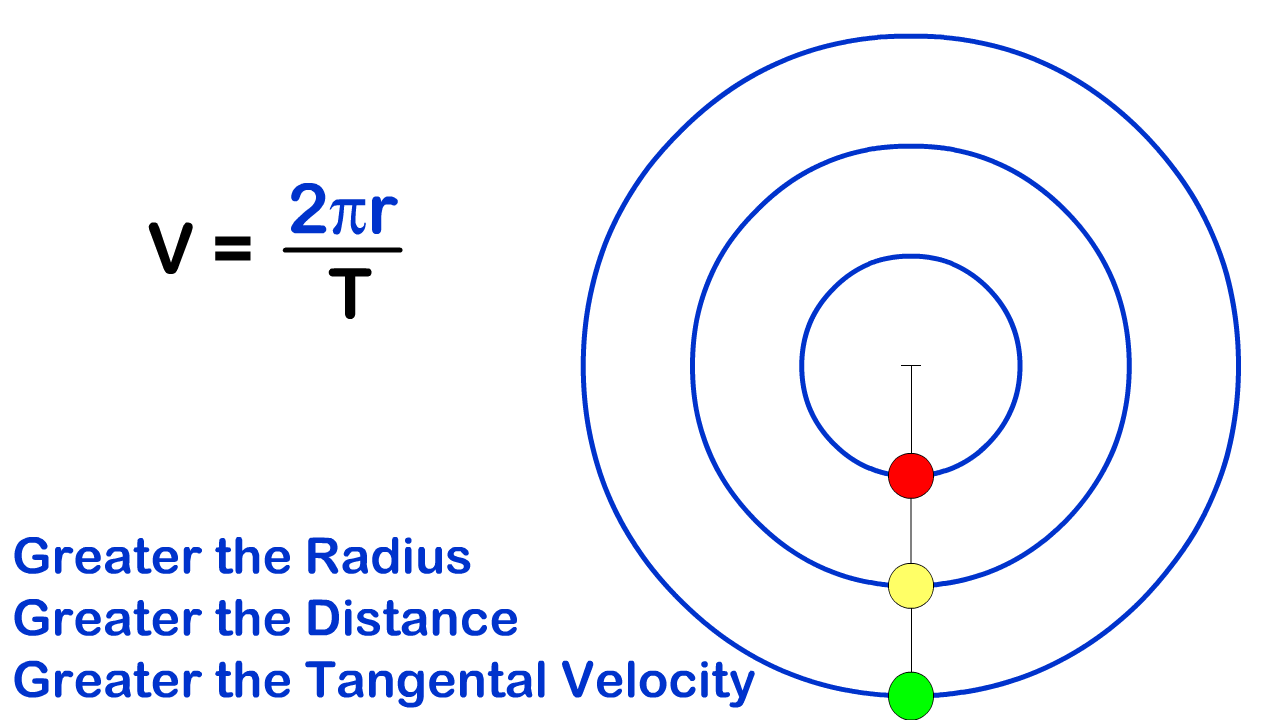
A greater radius gives an object a greater velocity when having the same period. You can analyze the equations in this unit along with any other using the rule of ones. Click here if you want a reminder on how to use this. Notice in the image that the fastest circle is green which is the furthest out and the slowest is red.

greater radius greater tangental velocity

Q14: What is the tangential velocity of a carnival ride that takes 5.0 seconds to go around in a circle with a radius of 20 meters?

Q15: Does tangential velocity increase or decrease when further away from the axis on a rotating object?

Q16: How much different is the tangential velocity if an object takes three times as much time to revolve around an object at the same radius?

Centripetal Force Example Problems

1. What would happen to the centripetal force required to keep an object going in a circle if the radius of a circle was doubled?

2. How many times the centripetal force would you have if a car slowed from 60 mph to 20 mph going around a curve?

3. Kent spins in his chair with a frequency of 0.5 Hz. What is the period of his spin?

F = 0.5 Hz

T = 1/f =1/0.5 = 2 s

(Click on the picture to enlarge it)

4. A record takes 1.3 s to make one complete rotation. An object on this record is 0.12 m from the center. What is its velocity?

5a. The pilot of a 60,500 kg jet plane is flying in circles whose radius is 5.00 x 10⁴m. It takes 1.8 x 10³ s to make one rotation. What is the velocity of the plane?